 equal
equal 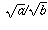 ?
?
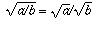 ,
except in the case in which a and b are both positive.
,
except in the case in which a and b are both positive.
If this surprises you, think about the question
Why shouldIf you were to try to convince someone of this, you'd have to start with the definition of what a "square root" is: it's a number whose square is the number you started with. So, from first principles, all that has to be true is thatequal
?
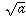 squared is a,
squared is a, 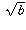 squared is b,
and
squared is b,
and  squared is a/b.
squared is a/b.
So, when you square  , you will get a/b, and when you
square
, you will get a/b, and when you
square 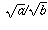 , you will also get a/b. That's all that the definition
of square root tells you.
, you will also get a/b. That's all that the definition
of square root tells you.
Now, the only way two numbers x and y can have the same square is if x = +/- y. So, what is true is that
but in general there's no reason it has to be,
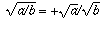 rather
than
rather
than 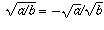 , unless
a and b are both positive: for then (because
by convention we take the positive square root) everything in the above
equation is positive, and that's why we obtain
, unless
a and b are both positive: for then (because
by convention we take the positive square root) everything in the above
equation is positive, and that's why we obtain 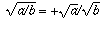 . But remember
it's only because everything is positive that we obtain it!
. But remember
it's only because everything is positive that we obtain it!
In our case, it is true that 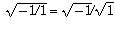 , but
, but
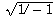 is
is  not
not 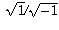 . The fallacy comes from
using the latter instead of the former.
. The fallacy comes from
using the latter instead of the former.
In fact, the whole proof really boils down to the fact that
(-1)(-1) = 1, so 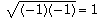 ,
but
,
but 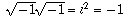 (not 1). The proof tried to
claim that these two were equal (but in a more disguised way where it
was harder to spot the mistake).
(not 1). The proof tried to
claim that these two were equal (but in a more disguised way where it
was harder to spot the mistake).
This fallacy is a good illustration of the dangers of taking a rule
from one context and just assuming it holds in another. When you first
learned about square roots you had never encountered complex numbers,
so the only objects that had sqare roots were positive numbers.
In this case, 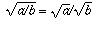 is always true, and you were probably taught it
as a "rule". But it is only a mathematical truth in that original
context, and fails to remain true after you extend the definition of
"square root" to allow the square roots of negative and complex numbers.
is always true, and you were probably taught it
as a "rule". But it is only a mathematical truth in that original
context, and fails to remain true after you extend the definition of
"square root" to allow the square roots of negative and complex numbers.