1.2 Constancy conditions, ${\mathcal K}_n/\delta{\mathcal K}_{n+1}$, and chord diagrams

As promised in the introduction, we study invariants of type n by studying their nth derivatives. Clearly, if V is of type n and $W=\partial^nV$, then $\partial W=0$ (``W is a constant''). Glancing at (2), we see that W descends to a linear functional, also called W, on ${\mathcal K}_n/\delta{\mathcal K}_{n+1}$:

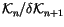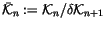
Definition 1.5   We call $\bar{\mathcal K}_n:={\mathcal K}_n/\delta{\mathcal K}_{n+1}$the space of ``n-symbols'' associated with the ladders in (2). (The name is inspired by the theory of differential operators, where the ``symbol'' of an operator is essentially its equivalence class modulo lower order operators. 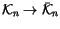The symbol is responsible for many of the properties of the original operator, and for many purposes, two operators that have the same symbol are ``the same''.) We denote the projection mapping ${\mathcal K}_n\to\bar{\mathcal K}_n$ that maps every singular knot to its symbol by $\pi$.

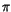
The following classical proposition (see e.g. [B-N1,Bi,BL,Go1,Go2,Ko1,Va1,Va2] identifies the space of n-symbols in our case:

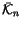
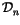
Proposition 1.6   The space $\bar{\mathcal K}_n$ of n-symbols for (2) is canonically isomorphic to the space ${\mathcal D}_n$ of n-chord diagrams, defined below. $\Box$

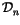
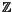
Definition 1.7   An n-chord diagram is a choice of n pairs of distinct points on an oriented circle, considered up to orientation preserving homeomorphisms of the circle. Usually an n-chord diagram is simply drawn as a circle with n chords (whose ends are the n pairs), as in the 5-chord example in Figure 3. The space ${\mathcal D}_n$ is the space of all formal ${\mathbb Z}$-linear combinations of n-chord diagrams.

  

Figure 3: A chord diagram.