Dror Bar-Natan: Classes: 2003-04: Math 157 - Analysis I:

(74)

Next: Solution of Term Exam 3 Previous: Class Notes for Thursday February 5, 2004

Term Exam 3

University of Toronto, February 9, 2004

This document in PDF: Exam.pdf

Solve the following 4 problems. Each is worth 25 points although they may have unequal difficulty. Write your answers in the space below the problems and on the front sides of the extra pages; use the back of the pages for scratch paper. Only work appearing on the front side of pages will be graded. Write your name and student number on each page. If you need more paper please ask the tutors. You have an hour and 50 minutes.

Allowed Material: Any calculating device that is not capable of displaying text.

Good Luck!

Problem 1. In a very condensed form, the definition of integration is as follows: For $f$ bounded on $[a,b]$ and $P: a=t_0<t_1<\dots<t_n=b$ a partition of $[a,b]$ set $m_i=\inf_{[t_{i-1},t_i]}f(x)$, $M_i=\sup_{[t_{i-1},t_i]}f(x)$, $L(f,P)=\sum_{i=1}^n m_i(t_i-t_{i-1})$ and $U(f,P)=\sum_{i=1}^n M_i(t_i-t_{i-1})$. Then set $L(f)=\sup_P L(f,P)$ and $U(f)=\inf_P U(f,P)$. Finally, if $U(f)=L(f)$ we say that ``$f$ is integrable on $[a,b]$'' and set $\int_a^b f=\int_a^b f(x)dx=U(f)=L(f)$.

From this definition alone, without using anything proven in class about integration, prove that the function $f$ given below is integrable on $[-1,1]$ and compute its integral $\int_{-1}^1f$:

$$f(x)=\begin{cases}0 & x\neq 0 1 & x=0. \end{cases}$$

Problem 2. Prove that the function

$$g(x):=\int_0^x\frac{dt}{1+t^2} + \int_0^{1/x}\frac{dt}{1+t^2}$$

is a constant function.

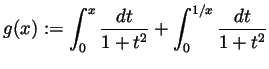
Problem 3. In class we have proven that a twice-differentiable function $f$ satisfying the equation $f''=-f$ is determined by $f(0)$ and $f'(0)$. Use this fact and the known formulas for the derivatives of $\cos x$ and $\sin x$ to derive a formula for $\cos(\alpha+\beta)$ in terms of $\cos\alpha$, $\cos\beta$, $\sin\alpha$ and $\sin\beta$.

Problem 4. The function $F$ is defined by $F(x):=x^x$.

1. Compute $F'(x)$ for all $x>0$.
2. Explain why $F(x)$ has a differentiable inverse for $x>\frac{1}{e}$.
3. Let $S$ be the inverse function of $F$ (with the domain of $F$ considered to be $(\frac{1}{e},\infty)$). Compute $S'(x)$ and simplify your result as much as you can. Your end result may still contain $S(x)$ in it, but not $S'$, $F$ or $F'$.

The generation of this document was assisted by L^ATEX2HTML.