Question Corner and Discussion Area
Question Corner and Discussion Area
Given a regular tetrahedral with a point in the center, find the angle formed from this center point to two corners (next to each other) in the tetrahedral. In chemistry terms you are actually proving that the the electrons in a tetrahedral shaped molecule are 109.5 degrees apart. No where in the proof however can you use the 109.5 degrees. This is the angle that you want to find.This souds like an assignment question. Our goal here is to help you understand mathematical concepts (especially ones that go beyond the standard curriculum), so we would prefer you to ask "Here is a question I have been given, and I would like to understand better how I should go apart tackling such-and-such a part of it . . . " rather than simply asking us the same question that was asked of you.
There are several ways to tackle this question. The simplest involves using vectors. Think about the four vectors from the centre of the tetrahedron to the four corners. You know several things about these vectors:
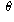 between any pair of vectors is the same, and from the
theory of dot products one has the fact that
between any pair of vectors is the same, and from the
theory of dot products one has the fact that
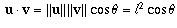 (where u and v are any two of
the vectors).
(where u and v are any two of
the vectors).
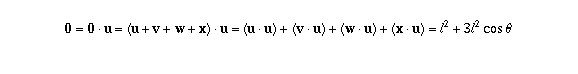
and therefore 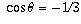 , which you can solve for
, which you can solve for 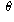 to get an answer of approximately 109.5 degrees.
to get an answer of approximately 109.5 degrees.
![]() Go backward to A Sequence Describing a Bouncing Ball
Go backward to A Sequence Describing a Bouncing Ball![]() Go up to Question Corner Index
Go up to Question Corner Index![]() Go forward to Counting Obtuse Triangles in an Inscribed Polygon
Go forward to Counting Obtuse Triangles in an Inscribed Polygon![]() Switch to text-only version (no graphics)
Switch to text-only version (no graphics)![]() Access printed version in PostScript format (requires PostScript printer)
Access printed version in PostScript format (requires PostScript printer)![]() Go to University of Toronto Mathematics Network
Home Page
Go to University of Toronto Mathematics Network
Home Page