



Next: 2. Background Material
Up: Finite Type Invariants by
Previous: Contents
1. Introduction
In the late 1980s, Vassiliev [Va1,Va2]
suggested to study knot invariants by studying the space of all
knots. The first new ingredient thus added is the notion of
``neighboring'' knots, knots that differ in only one crossing, and
the idea that one should study how knot invariants change in the
``neighborhood'' of a given knot. This idea led to a definition
of a certain class of knot invariants, now known as ``Vassiliev''
or ``finite type'' invariants. By now more than 350 papers have been
written on the subject; see [B-N2]. Vassiliev's original
definition (independently discovered by Goussarov [Go1,Go2] at roughly the same time) was formalized
in a different way by Birman and Lin [BL],
and later re-interpreted by analogy with multi-variable calculus by
Bar-Natan [B-N1].
The basic idea in Bar-Natan [B-N1] was that
differences are ``cousins'' of derivatives, and hence one should
think of the difference between the values of a knot invariant
V on two neighboring knots as a ``derivative'' of the original
invariant. Repeating this, we find that iterated differences (as
considered by [Va1,Va2,Go1,Go2,BL,B-N1])
of values of V in the neighborhood of some knot should be thought of
as multiple derivatives. A ``Vassiliev'' or ``finite type'' invariant
of type m is then the analog of a polynomial -- an invariant whose
m+1st derivatives, or m+1st iterated differences, vanishes.
Clearly, these ideas are very general, and knots (and even topology in
general) are just a particular case. Whenever an appropriate notion of
``neighborhood of an object'' exists, one can talk about finite type
invariants of such objects. This leads to many different ``species''
of finite type invariants. Let us mention just a few:
- The usual notion of nearness of knots, knots that differ at only
a single crossing, leads to the usual Vassiliev invariants.
- Similarly, one can define ``Vassiliev'' invariants of braids, links,
tangles, knotted graphs, etc.
- Goussarov [Go3] has also an alternative
notion of a neighborhood of a knot (or link), defined by
``interdependent modifications''. This notion leads to a different
(though at the end, equivalent) theory of finite type invariants of
knots and links.
- Two algebraically split links (links whose linking numbers all vanish)
can be considered neighboring if they differ by the simultaneous flip of
two opposite crossings between two given components (such a double
flip preserves linking numbers, whereas a single flip doesn't). See
Figure 1. This leads to a little known but probably
interesting theory of finite type invariants of algebraically split
links.
Figure 1:
A move that preserves linking numbers.
![\begin{figure}
\begin{displaymath}
\if ny
\smash{\makebox[0pt]{\hspace{-0.5in...
...draws/ASMove.tex }
\hspace{-1.9mm}
\end{array} \end{displaymath}
\end{figure}](img7.gif) |
- Several authors [] have considered several notions of finite type
invariants of plane curves.
- Ohtsuki [Oh1] considers two integral
homology spheres to be neighboring (roughly) if they differ by a single
surgery. This leads to a notion of finite type invariants of integral
homology spheres. Several variants of his definition were considered
in [Ga1,GL1,GO1,GL2,GO2,Ga2,GL3]
A finite type theory automatically comes bundled with several spaces
that play a significant role in it. In the best known case of knots,
these are the spaces of chord diagrams, 4T relations, weight systems,
chord diagrams modulo 4T relations, relations between 4T relations,
and a few lesser known spaces that should probably be better known. There
is a ``general theory of finite type invariants'', defined in terms of
these spaces, in which one attempts to classify finite type invariants by
first classifying their potential mth derivatives, and then by studying
which of those potential derivatives can actually be integrated to an
honest invariant. I should say that though this ``general theory'' is
rather small, it is also rather interesting (with the most interesting
parts developed by M. Hutchings [Hu] and
private communication), and insufficiently well known even in the
case of the usual finite type invariants of knots.
The purpose of this paper is twofold:
- 1.
- To state (and propagate) this general theory of finite type
invariants of anything. Namely, to construct, name, and study
the relationships between those spaces that come automatically
with every finite type theory, especially from the perspective of
the integration theory of ``weight systems''. We first do it in
Section 1 on a well known example, the original finite
type theory of knots. We then extract some general features from this
example and give them general names; this is done in the rather short
Section 2.
- 2.
- To list many of the currently known finite type theories, and
figure out (to the degree that is now possible) what these associated
spaces are on a species by species basis. Our list takes the form of a
``classification''1.
The top subdivision is into the classes of ``Knotted Objects'',
``3-Manifolds'' and ``Plane Curves'', and these classes are
described in Sections 4, 5
and 6, respectively.
Acknowledgement: I wish to thank Mike Hutchings for the inspiration
to write this paper and for telling me his ideas about integration
theory. I also wish to thank E. Appleboim and H. Scolnicov for many
ideas and conversations.
Footnotes
- ... ``classification''1
-
classification (klàs´e-f
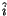 -k
-k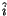 ´shen), in biology,
the systematic categorization of organisms. One aim of modern
classification, or systematics, is to show the evolutionary
relationships among organisms. The broadest division of organisms
is into kingdoms, traditionally two-Animalia (animals) and Plantae
(plants). Widely accepted today are three additional kingdoms:
the Protista, comprising protozoans and some unicellular algae;
the Monera, bacteria and blue-green algae; and the Fungi. From
most to least inclusive, kingdoms are divided into the following
categories: phylum (usually called division in botany), class, order,
family, genus, and species. The species, the fundamental unit of
classification, consists of populations of genetically similar,
interbreeding or potentially interbreeding individuals that share
the same gene pool (collection of inherited characteristics whose
combination is unique to the species). Copyright ©1995
by Columbia University Press. All rights reserved.
´shen), in biology,
the systematic categorization of organisms. One aim of modern
classification, or systematics, is to show the evolutionary
relationships among organisms. The broadest division of organisms
is into kingdoms, traditionally two-Animalia (animals) and Plantae
(plants). Widely accepted today are three additional kingdoms:
the Protista, comprising protozoans and some unicellular algae;
the Monera, bacteria and blue-green algae; and the Fungi. From
most to least inclusive, kingdoms are divided into the following
categories: phylum (usually called division in botany), class, order,
family, genus, and species. The species, the fundamental unit of
classification, consists of populations of genetically similar,
interbreeding or potentially interbreeding individuals that share
the same gene pool (collection of inherited characteristics whose
combination is unique to the species). Copyright ©1995
by Columbia University Press. All rights reserved.




Next: 2. Background Material
Up: Finite Type Invariants by
Previous: Contents
Dror Bar-Natan
2000-03-19