Dror Bar-Natan: Classes: 2003-04: Math 1350F - Knot Theory:

(31)

Next: Class Notes for Thursday October 23, 2003 Previous: Class Notes for Tuesday October 21, 2003

Homework Assignment 6: Deframing

Assigned Thursday October 23; due Thursday October 30 in class.

This document in PDF: HW.pdf

Required reading. Sections 2 and 3 of my paper On the Vassiliev Knot Invariants.

Let $\Theta:{\mathcal A}\to{\mathcal A}$ be the multiplication operator by the chord diagram $\theta$, and let $\partial_\theta=\frac{d}{d\theta}$ be the adjoint of multiplication by $W_\theta$ on ${\mathcal A}^\star$, where $W_\theta$ is the obvious dual of $\theta$ in ${\mathcal A}^\star$. Let $P:{\mathcal A}\to{\mathcal A}$ be defined by

$$P=\sum_{n=0}^\infty \frac{(-\Theta)^n}{n!}\partial_\theta^n.$$

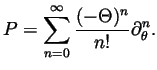
The following assertions can be verified:

1. $\left[\partial_\theta, \Theta\right]=1$, where $1:{\mathcal A}\to{\mathcal A}$ is the identity map and where $[A,B]:=AB-BA$ for any two operators.

2. $P$ is a degree 0 operator; that is, $\deg Pa=\deg a$ for all $a\in{\mathcal A}$.

3. $\partial_\theta$ satisfies Leibnitz' law: $\partial_\theta(ab)=(\partial_\theta a)b+a(\partial_\theta b)$ for any $a,b\in{\mathcal A}$.

4. $P$ is an algebra morphism: $P1=1$ and $P(ab)=(Pa)(Pb)$.

5. $\Theta$ satisfies the co-Leibnitz law: $\Box\circ\Theta=(\Theta\otimes 1+1\otimes\Theta)\circ\Box$ (why does this deserve the name ``the co-Leibnitz law''?).

6. $P$ is a co-algebra morphism: $\eta\circ P=\eta$ (where $\eta$ is the co-unit of ${\mathcal A}$) and $\Box\circ P=(P\otimes P)\circ\Box$.

7. $P\theta=0$ and hence $P\langle\theta\rangle=0$, where $\langle\theta\rangle$ is the ideal generated by $\theta$ in the algebra ${\mathcal A}$.

8. If $Q:{\mathcal A}\to{\mathcal A}$ is defined by
   $$Q = \sum_{n=0}^\infty \frac{(-\Theta)^n}{(n+1)!}\partial_\theta^{(n+1)}$$
   then $a=\theta Qa+Pa$ for all $a\in{\mathcal A}$.

9. $\ker P=\langle\theta\rangle$.

10. $P$ descends to a Hopf algebra morphism ${\mathcal A}^r\to{\mathcal A}$, and if $\pi:{\mathcal A}\to{\mathcal A}^r$ is the obvious projection, then $\pi\circ P$ is the identity of ${\mathcal A}^r$. (Recall that ${\mathcal A}^r={\mathcal A}/\langle\theta\rangle$.)

11. $P^2=P$.

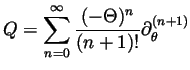
To be handed in. Verify assertions 4, 5, 7 and 11 above.

Recommended for extra practice. Verify all the other assertions above.

Idea for a good deed. Prepare a beautiful TEX writeup (including the motivation and all the details) of the solution of this assignment for publication on the web. For all I know this information in this form is not available elsewhere.

The generation of this document was assisted by L^ATEX2HTML.