Assignment 7 Solutions

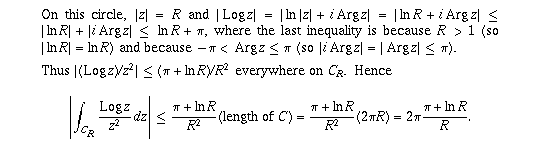
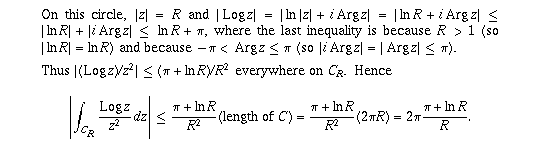
Page 116 #2

Page 116 #4

(a) f(z) is analytic except at z=3. The point z=3 lies outside the contour C. This means f(z) is analytic everywhere on and inside C, so the Cauchy-Goursat Theorem guarantees that the contour integral of f(z) over C is zero.

(c) f(z) is analytic except where 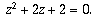Solving this using the quadratic equation gives 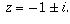These points have modulus 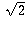and hence lie outside the contour C. This means f(z) is analytic everywhere on and inside C, so the Cauchy-Goursat Theorem guarantees that the contour integral of f(z) over C is zero.

Page 117 #6 Let D be a circle centred at 2+i, oriented positively, and of small enough radius that it is contained entirely within the rectangle C. For example, you could take D to be of radius 1/2 as illustrated below.

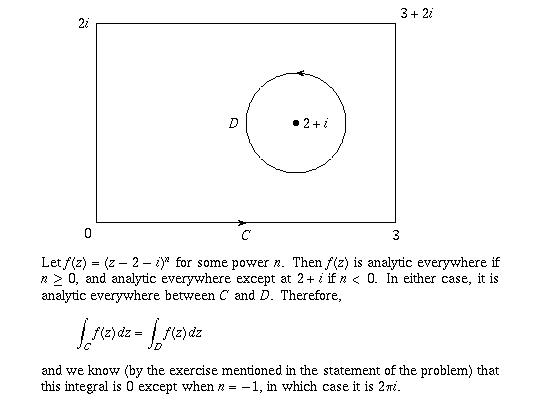
Page 117 #8

Page 118 #9

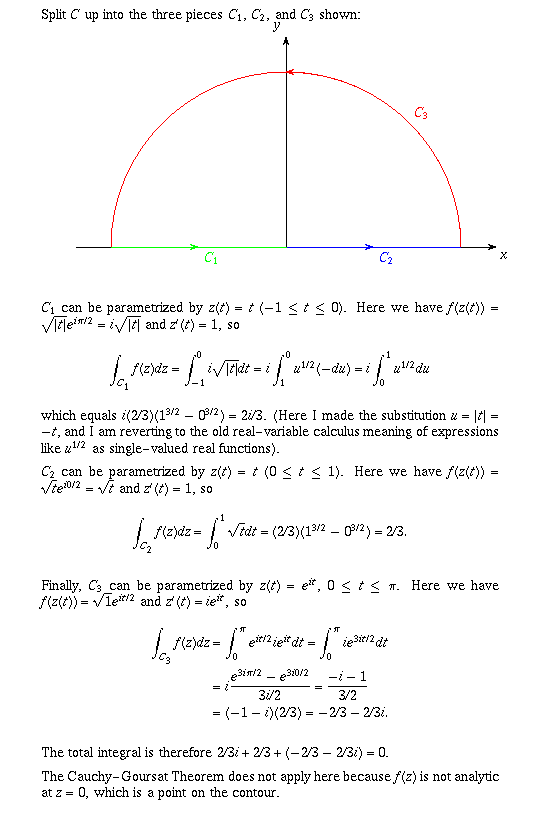
Go backward to Assignment 7 (due Thursday November 6th)
Go up to Contents
Go forward to Assignment 8 (due Thursday November 20th)
Switch to text-only version (no graphics)