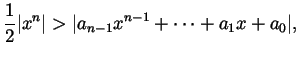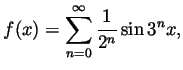Dror Bar-Natan: Classes: 2002-03: Math 157 - Analysis I:

(80)

Next: Wang Ying's Solution of Homework Assignment 8 Previous: Class Notes for the Week of October 21 (8 of 8)

Homework Assignment 8

Assigned Tuesday October 29; due Friday November 8, 2PM at SS 1071

this document in PDF: HW08.pdf

Required reading

All of Spivak Chapter 9.

To be handed in

From Spivak Chapter 9: 1, 9, 15, 23.

Recommended for extra practice

From Spivak Chapter 9: 8, 11, 21, 28.

Also, let 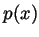$p(x)$ be the polynomial 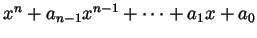$x^n+a_{n-1}x^{n-1}+\dots+a_1x+a_0$. Now that we know that for 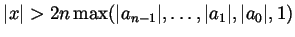$\vert x\vert>2n\max(\vert a_{n-1}\vert,\dots,\vert a_1\vert,\vert a_0\vert,1)$ we have that

$$\frac12 \vert x^n\vert > \vert a_{n-1}x^{n-1}+\dots+a_1x+a_0\vert,$$

complete the proof of the following

Theorem.

• If $n$ is odd then the equation 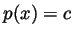$p(x)=c$ has a root for any value of $c$.
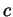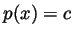• If $n$ is even then there is some constant $c_0$ so that the equation $p(x)=c$ has no roots for 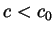$c<c_0$, has at least one root for 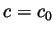$c=c_0$ and at least two roots for $c>c_0$.

Just for fun

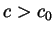Write a computer program that will allow you to draw the graph of the function

$$f(x)=\sum_{n=0}^\infty \frac{1}{2^n}\sin 3^nx,$$

and will allow you to zoom on that graph through various small ``windows''. Use your program to convince yourself that 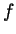$f$ is everywhere continuous but nowhere differentiable. The best plots will be posted on this web site! (Send pictures along with window coordinates by email to drorbn@math.toronto.edu).

The generation of this document was assisted by L^ATEX2HTML.