| Dror Bar-Natan: Classes: 2002-03: Math 157 - Analysis I: | (285) |
Next: Solution of the Final Exam
Previous: Sample Final Exam |
Solve the following 6 problems. Each is worth 20 points although they may have unequal difficulty, so the maximal possible total grade is 120 points. Write your answers in the space below the problems and on the front sides of the extra pages; use the back of the pages for scratch paper. Only work appearing on the front side of pages will be graded. Write your name and student number on each page. If you need more paper please ask the presiding officers. This booklet has 12 pages.
Duration. You have 3 hours to write this exam.
Allowed Material: Any calculating device that is not capable of displaying text.
Problem 1. Let ![]() and
and ![]() denote functions defined on
some set
denote functions defined on
some set ![]() .
.
Problem 2. Sketch the graph of the function
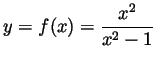 . Make sure that your graph clearly
indicates the following:
. Make sure that your graph clearly
indicates the following:
Problem 3. Compute the following integrals:
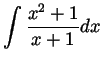 .
.
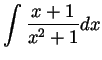 .
.
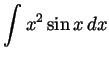 .
.
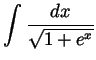 .
.
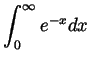 .
.
Problem 4. Agents of the
CSIS have secretly developed a
function ![]() that has the following properties:
that has the following properties:
Problem 5.
Problem 6. Prove that the complex function
![]() is everywhere continuous but nowhere differentiable.
is everywhere continuous but nowhere differentiable.