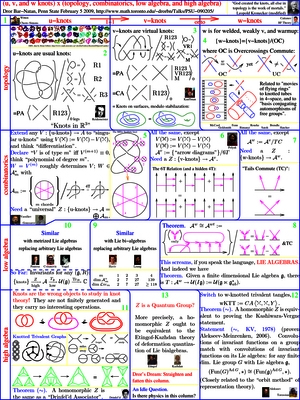
Abstract. My subject is a Cartesian product. It runs in three parallel columns - the u column, for usual knots, the v column, for virtual knots, and the w column, for welded, or weakly virtual, or warmup knots. Each class of knots has a topological meaning and a "finite type" theory, which leads to some combinatorics, somewhat different combinatorics in each case. In each column the resulting combinatorics ends up describing tensors within a different "low algebra" universe - the universe of metrized Lie algebras for u, the richer universe of Lie bialgebras for v, and for w, the wider and therefore less refined universe of general Lie algebras. In each column there is a "fundamental theorem" to be proven (or conjectured), and the means, in each column, is a different piece of "high algebra": associators and quasi-Hopf algebras in one, deformation quantization à la Etingof and Kazhdan in the second, and in the third, the Kashiwara-Vergne theory of convolutions on Lie groups. Finally, u maps to v and v maps to w at topology level, and the relationship persists and deepens the further down the columns one goes.
The 12 boxes in this product each deserves its own talk, and the few that are not yet fully understood deserve a few further years of research. Thus my talk will only give the flavour of a few of the boxes that I understand, and only hint at my expectations for the contents the (2,4) box, the one I understand the least and the one I wish to understand the most.