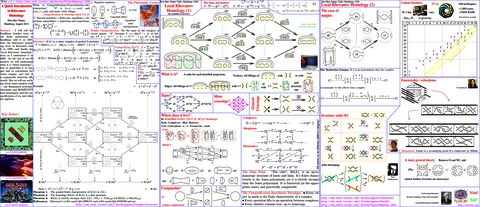
1. A Quick Introduction to Khovanov Homology
Abstract. I will tell the Kauffman bracket story of the Jones polynomial as
Kauffman told it in 1987, then the Khovanov homology story as
Khovanov told it in 1999, and finally the "local Khovanov homology"
story as I understood it in 2003. At the end of our 90 minutes we will
understand what is a "Jones homology", how to generalize it to tangles and to
cobordisms between tangles, and why it is computable relatively efficiently.
But we will say nothing about more modern stuff - the Rasmussen invariant,
Alexander and HOMFLYPT knot homologies, and the categorification of
sl2 and other Lie algebras.
Talk video. 
.
Handout: KH.html, KH.pdf,
KH.png.
Sources: KH.zip.
Pensieve: 2012-08
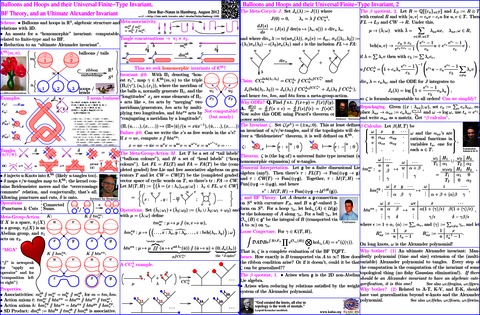
2. Balloons and Hoops and their Universal Finite
Type Invariant, BF Theory, and an Ultimate Alexander Invariant
Abstract. Balloons are two-dimensional spheres. Hoops are one
dimensional loops. Knotted Balloons and Hoops (KBH) in 4-space behave
much like the first and second fundamental groups of a topological space
- hoops can be composed like in
π1, balloons
like in
π2, and hoops "act" on balloons as
π1 acts on
π2. We will
observe that ordinary knots and tangles in 3-space map into KBH in
4-space and become amalgams of both balloons and hoops.
We give an ansatz for a tree and wheel (that is, free-Lie and
cyclic word) -valued invariant ζ of KBHs in terms of the said
compositions and action and we explain its relationship with finite type
invariants. We speculate that ζ is a complete evaluation of
the BF topological quantum field theory in 4D, though we are not sure
what that means. We show that a certain "reduction and repackaging"
of ζ is an "ultimate Alexander invariant" that contains the
Alexander polynomial (multivariable, if you wish), has extremely good
composition properties, is evaluated in a topologically meaningful
way, and is least-wasteful in a computational sense. If you believe in
categorification, that's a wonderful playground.
