

Abstract. Let there be scones! Our view of knot theory is biased in favour of pancakes.
Technically, if $K$ is a 3D knot that fits in volume $V$ (assuming fixed-width yarn), then its projection to 2D will have about $V^{4/3}$ crossings. You'd expect genuinely 3D quantities associated with $K$ to be computable straight from a 3D presentation of $K$. Yet we can hardly ever circumvent this $V^{4/3}\gg V$ "projection fee". Exceptions probably include the hyperbolic volume and certainly include finite type invariants (as we shall prove). But knot polynomials and knot homologies seem to always pay the fee.
Handout: YarnBallKnots.pdf
Annotated Slides: YarnBallKnots@.pdf
 Talk Video. (Also @YouTube).
Talk Video. (Also @YouTube).
URL: http://drorbn.net/fi20.
Aborted Plan:
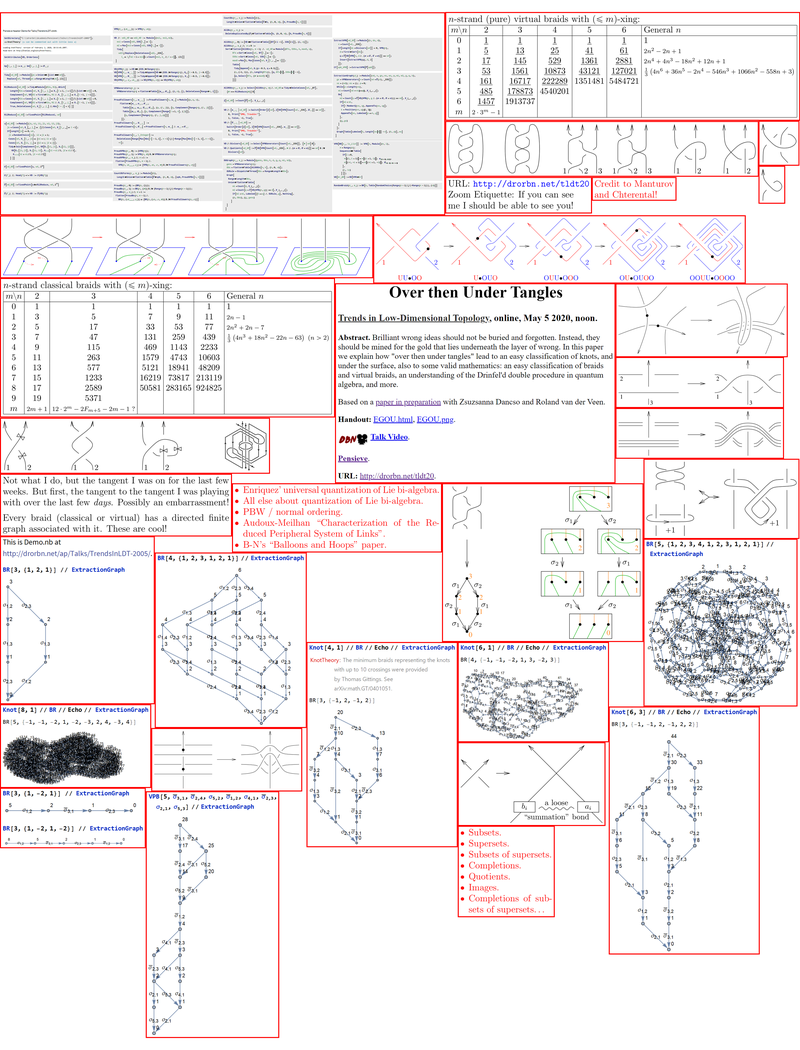
Abstract. Brilliant wrong ideas should not be buried and forgotten. Instead, they should be mined for the gold that lies underneath the layer of wrong. In this talk I will explain how "over then under tangles" lead to an easy classification of knots and to a proof that all knots are trivial, and under the surface, also to some valid mathematics: an easy classification of braids and virtual braids, a lovely assignments of graphs to braids, an understanding of the Drinfel'd double procedure in quantum algebra, and more.
Based on a paper with Zsuzsanna Dancso and Roland van der Veen.