| © | << < ? > >> | Dror Bar-Natan: Talks: | Links:
AM
AT
CS
Dal
Demo
KBH
KV
Lake
WKO1
WKO2
WKO
X
ZD
inf
mac
vX
Handout booklet: BannoyeBooklet.pdf |
Abstract. I will describe a general machine, a close cousin of Taylor's theorem, whose inputs are topics in topology and whose outputs are problems in algebra. There are many inputs the machine can take, and many outputs it produces, but I will concentrate on just one input/output pair. When fed with a certain class of knotted 2-dimensional objects in 4-dimensional space, it outputs the Kashiwara-Vergne Problem (1978, solved Alekseev-Meinrenken 2006, elucidated Alekseev-Torossian 2008-2011), a problem about convolutions on Lie groups and Lie algebras.
Handout:
KVT.html,
KVT.pdf,
KVT.png.
Sources: pensieve.
Talk video:

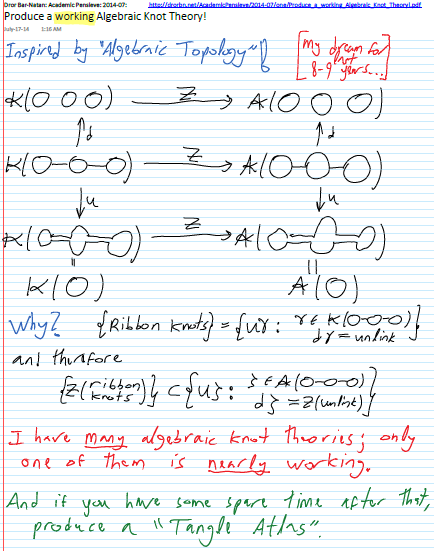
Abstract. I will describe some very good formulas for a (matrix plus scalar)-valued extension of the Alexander polynnomial to tangles, then say that everything extends to virtual tangles, then roughly to simply knotted balloons and hoops in 4D, then the target space extends to (free Lie algebras plus cyclic words), and the result is a universal finite type of the knotted objects in its domain. Taking a cue from the BF topological quantum field theory, everything should extend (with some modifications) to arbitrary codimension-2 knots in arbitrary dimension and in particular, to arbitrary 2-knots in 4D. But what is really going on is still a mystery.
Handout:
GoodFormulas.html,
GoodFormulas.pdf,
GoodFormulas.png.
Sources: pensieve.
Talk video: