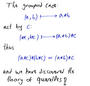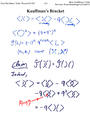In an article bearing a similar title1, Eugene Wigner once wondered about the numerous and unexpected ways in which mathematics comes into play in the natural sciences. While our topic and claims are much humbler, we find ourselves puzzled by the unreasonable affinity of knot theory and certain parts of algebra. It figures that knot theory is related to 3-dimensional topology. But why on earth should the mundane study of tangled shoelaces and unwieldy piles of seamen's rope be related to the elegance and sophistication of the likes of Lie algebras, 6j-symbols and homological algebra?
I'll start with the vague but fundamental relationship between knots, quandles and Lie algebras, which in my opinion is one of the quickest ways to notice that there's something algebraically deep about knots. I'll wave my hands about the deep relations via quantum field theory and quantum groups, talk a little about the Kauffman bracket and the Jones polynomial, and say a few words about Khovanov's categorification of the Jones polynomial2,3. That last topic is hardly understood; perhaps because it is the one place where knot theory is a bit ahead of its algebra.
1 Eugene Wigner, The Unreasonable Effectiveness of Mathematics in the Natural Sciences, Communications in Pure and Applied Mathematics 13-I (1960).
2 Mikhail Khovanov, A categorification of the Jones polynomial, arXiv:math.QA/9908171.
3 Dror Bar-Natan, On Khovanov's categorification of the Jones polynomial, arXiv:math.GT/0201043.
