It's a bit tough to write a research proposal for research that will take place more than a year from now. In the optimistic scenario, we will finish everything that's on our desks right now, and be ready to sail on into the horizon. In the pessimistic scenario, we will still be struggling with the details of a paper we started 10 years ago. In the most realistic scenario, we will be pushing our understanding of emergent knots (and other knotted objects: links, braids, tangles, etc.) in a pole dancing studio.
Grant proposals are often written as if they describe a victory parade. "The principal investigator will march fromA toB toC collecting trophies along the way". I hope this one is written differently. There is a single "!" in it, and it is in quotes. There are plenty of "?" in it. Each one represents a dream. A question that I plan to study and that I hope I have the tools to address and to elucidate, if not resolve. Will the NSERC help?
Summary of Proposal. I'm considered an expert on Knot Theory, yet I don't understand knot theory at all. From a certain perspective, Knot Theory is the study of some silly combinatorial objects, that are considered modulo equally silly relations. My intuition as a student told me it must be a shallow topic, and there's still a remnant of that intuition in me.Yet time after time this intuition is proven wrong and instead of shallow, Knot Theory is very deep. So much so, that Knot Theory sometimes serves to validate that other topics are interesting: if it has applications to Knot Theory, it must be good. (Historically, number theory's raison d'être had been similar; recently cryptography became a further bonus).
My plan over the grant period would be to continue to use knot theory as an excuse and as a benchmark to study several other topics
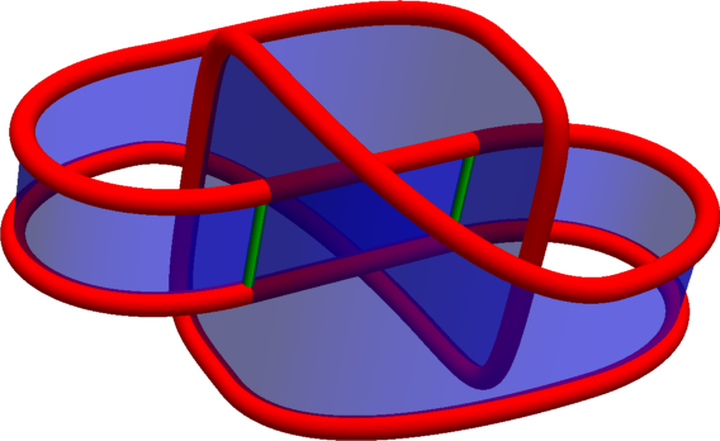
Beyond their knottedness, some knots may or may not have extra properties, like "being the boundary of a surface with certain allowed self-intersections" (picture on right, made 2017), or "being the boundary of a 2-dimensional disk in a 4-dimensional ball". Via "surgery", knots can be used to describe various alternative 3-dimensional and 4-dimensional spaces: roughly, you start with the standard Euclidean space, take a knotted tube out, put it back in in a different way, and you get an alternative 3D or 4D space. Thus it turns out that studying knots and their properties is a key to all of low dimensional topology. Sometimes knot invariants shed light on these extra properties that low dimensional topologists care about.
I aim to find and study strong knot and tangle invariants that behave well under knot/tangle operations (and hence they may shed light on topological properties that are definable in terms of these operations), and that are computable in polynomial time (and hence can be computed even on very large knots). The invariants I study come in a slightly non-standard way from quantum groups. I dream that one day I will understand quantum groups at a deeper level, and I do my best to realize this dream.
Achievement 10. My series of papers with R. van der Veen (Proc. Amer. Math. Soc. 147 (2019) 377-397, arXiv:2109.02057, and in preparation) represent the achievement I'm the most proud of, though it is not yet recognized by others. By using "solvable approximations of Lie algebras" and a fully algebraic version of Gaussian perturbation theory we construct a strong (in a measurable sense) poly-time computable (and hence real-life computable) invariant of knots that has good algebraic properties (in a quantifiable sense, leading to topological applications).
Perhaps a footnote isn't the right place to raise a major issue, yet I believe we don't genuinely understand the relationship between quantum groups and knot theory: if we know quantum groups we know how to make knot invariants, but the relationship should also go the other way. One has to be able to start with "we want knot invariants" and be lead to the specific formulas appearing in the quantizations of semi-simple Lie algebras. The narrative for the latter direction, as it stands now, is far from complete. It is hard to expect that our work in itself will change this situation. Yet we must hover around these issues if we ever want to fully understand them, and this we do.
Knots are as old as mankind. The first mathematical paper which has mentioned knots is "Remarques sur les problèmes de situatio" by A.T. Vandermonde in 1771. Since then, mathematicians try to solve the main problem in knot theory: for two given knots in 3-space, decide in an effective way whether or not they are isotopic, i.e. can be deformed one into the other in a continuous way?
Press Release. We study knot-like objects in 2-dimensional universes (where they are just curves drawn on surfaces), in 3-dimensional universes (where they are exactly what we ordinarilyy call knots) and in 4-dimensional universes (where they are two-dimensional surfaces knotted inside 4-dimensional spaces). In each case the study leads to a problem in algebra: a problem in Lie theory in dimension 4, a problem regarding non-associativity in dimension 3, and again a problem in Lie theory in dimension 2; the same one that first arose in dimension 4.We understand how the solution to the "3" problem (the celebrated "Drinfel'd Associators") can be used to solve the "2" and the "4" problems. But the "2" and the "4" problems are the same, so there must be a direct relationship between our knot-like objects in 2-dimensions and in 4-dimensions. We plan to find it!
Summary of Proposal. Totally by definition, once in a lifetime, a researcher is working on his personal best project. For me this is now, and I'm very excited about it. Let me explain.Here and there math has immense philosophical value or beauty to justify the effort. Yet everyday math is mostly about, or should be about, "doing useful things". Deciding if A has property B, counting how many C's satisfy D, computing E. When A and B and C and D and E are small, we do the computations on the back of an envelope and write them as "Example 3.14" in some paper. But these are merely the demos, and sooner or later we worry (or ought to worry) about bigger inputs. I'm more aware then most mathematicians (though perhaps less than many computer scientists), how much the complexity of obtaining the solution as a function of the size of the inputs matters. Hence I firmly believe that incomputable mathematics is intrinsically less valuable than computable mathematics (allowing some exceptions for philosophical value and/or beauty), and that within computable mathematics, what can be computed in linear time is generally more valuable than what can be computed in polynomial (poly-) time, which in itself is more valuable than what can be computed in exponential (exp-) time, which in itself is more valuable than what can be computed just in theory.
I've always been an exp-time mathematician. Almost everything I've worked on, finite-type invariants and invariants of certain 3-manifolds, categorification, matters related to associators and to free Lie algebras, etc., boils down to computable things, though they are computable in exp-time.
My current project (joint with Roland van der Veen and continuing Lev Rozansky and Andrea Overbay) is poly-time, which puts it ahead of everything else I have done. IMHO it is also philosophically interesting and beautiful, but I'm biased. ...
We hope to bring together a number of experts working on "expansions" and a number of experts working on "invariants" in the hope that the two groups will learn from each other and influence each other. "Expansions" are solutions of a certain type of intricate equations within graded spaces often associated with free Lie algebras; they include Drinfel'd associators, solutions of the Kashiwara-Vergne equations, solutions of various deformation quantization problems, and more. By "invariants" we refer to quantum-algebra-inspired invariants of various objects within low dimensional topology; these are often associated with various semi-simple Lie algebras. The two subjects were born together in the early days of quantum group theory, but have to a large extent evolved separately. We believe there is much to gain by bringing the two together again.
The only poly-time [knot] invariants we now know are the Alexander polynomial and finite type invariants. The Alexander polynomial can be computed in about n4 operations (where n is any reasonable measure of the complexity of the knot). Each coefficient of the Alexander polynomial is a numerical invariant, and hence there are infinitely many numerical invariants that can be computed in about n4 time. Finite type invariants can also be computed in poly-time, but there are only finitely many of them below any nd time bound. So Alexander stands out as an anomaly or a miracle: an infinite garden within a scarcely planted desert.This informal picture, of an Alexander miracle in a great desert, is so unusual it must either be proven or refuted. I plan to do the latter by constructing a few new poly-time computable polynomial-valued knot invariants.
If the natural numbers 1,2,3,... are the simplest and purest of algebra, knots are the simplest and purest of "topology" - that part of mathematics which studies what cannot be changed by a gentle morphing, and can only be modified by abruptly cutting and tearing. Knots are bent and bending pieces of string in our usual 3-dimensional space. Sailors and mountain climbers have been using them since ever, and mathematicians have been studying them for almost as long.The topic of our workshop is "virtual knots". A relatively recent generalization of the classical notion of knots, which sometimes represents ordinary knots and sometimes represents knots in 4-dimensional space, yet in general lives in no dimension in particular. One reason we care about virtual knots is because of their deep relationship with algebra in general and with "quantum algebra" in particular.
I study 1-knots and 2-knots. 1-Knots are exactly what you think they are - they are pieces of 1-dimensional strings placed in "knotted ways" inside 3-dimensional space. How can you prove that such a piece of string is really knotted and cannot be undone? 2-Knots are exactly what you'd think they are if you were living in a 4-dimensional space. They are 2-dimensional pieces of cloth, placed in "knotted ways" inside 4-dimensional space. What?? Do these things at all exist? What's 4D anyway? These, I guess, are the questions with which my subject started. By now they are settled, and the questions go much deeper, into the relationship between 1-knots and 2-knots and many topics around them, including algebra and geometry and (what?) quantum field theory.The first picture on the right is a 1-knot. The second is a 2-knot. Because of the physical limitations of your screen (and mine), it appears to be a surface in 3-dimensional space. The fourth dimension is coded using colours, much as colours often code height in 2-dimensional topographical maps. Red means "high" on the fourth coordinate, and blue means low. The third picture is the same as the second, except cut in half, to make the "inside" visible.
Impact. My main project on the v-column, if successful, will radically change the way deformation quantization is viewed, from a theory of deformations of certain algebraic objects, to a theory of "homomorphic expansions" of certain topological objects. I believe it will lead to a radical re-interpretation of the theory of quantum groups. My secondary project may lead to a wide-ranging extension of the most successful knot invariant, the Alexander polynomial, in several directions. In addition, I hope to continue to impact how math is done, presented, and disseminated, by being and remaining at the head of the technological curve.
Very simple. As should be clear from my "Statement of Recent Work", Much is done, much remains to do, and little is written. I plan to spend my already-approved half-sabbatical mostly in Toronto, mostly writing. I hope to spend the Simons half-sabbatical writing even further and working with Anton Alekseev in Geneva (also taking excursions to visit Benjamin Enriquez in Strasbourg), mostly, I hope, completing the "v" column of my "Statement of Recent Work", in which many mysteries still remain.
There is a machine that I like to call "projectivization", which takes certain problems in knot theory and related topics, mostly related to finite type invariants and their interaction with various topological operations, and turns them into problems in graded algebra, often related to Drinfel'd associators and other quantum-group-related constructs. A surprising amount of algebra is in the image of the projectivization machine: associators (web/nat, web/ktgs), the Grothendieck-Teichmuller group (web/gt), the Kashiwara-Vergne conjecture (web/wko), and more. One of my primary goals will be to show that the same is true for the work of Etingof and Kazhdan on the quantization of Lie bialgebras. In some sense this will mean that the theory of quantum groups not only has applications to topology, but in fact, _it is_ topology.
I believe math is too deep. Rather than making it deeper, a better use of my time would be to make some deep ends easier and more accessible. I believe math is too abstract, or at least appears to be too abstract, for much of what may be computed hardly ever is. Thus, whenever I can, I code. Yet I have sinned a few times and written on deep math that was not accompanied with programs. I usually work on knot theory and its surprising relationship with algebra, geometry and quantum field theory. I got my Ph.D. at Princeton, did time at Harvard, Hebrew U., Berkeley and MSRI, and I now work at the University of Toronto. Practically everything I've ever done (including even this paragraph) can be found somewhere on my website, starting at http://www.math.toronto.edu/~drorbn/.
I'll start with the question, and then try to put some meaning into it.
Question. Sometimes a bit of algebra turns out to be a bit of topology, in disguise. Is that true for the theory of quantum groups? [...]
[...] Number theory just seems to be related to everything.
Likewise, though on a smaller scale, many knot theorists such as myself care little about shoelaces, yet care a lot about the unexpected ways by which the study of knotted shoelaces is intricately and deeply related to such a priori remote subjects as 3-dimensional manifolds, hyperbolic geometry, quantum field theory, differential geometry, Lie theory and representation theory, quantum algebra, combinatorics, homological algebra and sophisticated algorithmics.
What about Khovanov homology? I made significant contributions to the highly fashionable subject of Khovanov homology (in fact, while Khovanov is definitely the father of the field, I share the credit for making it fashionable...). Yet at the moment I don't feel mature enough to study this topic any further. I'd rather "categorify" knot invariants only after I properly understand the "algebra" on which they ought to be defined (in the sense of my first project above). And how can I even start categorifying other aspects of the theory of quantum groups, when in my opinion this theory in itself is so poorly understood (at least in the sense of my second project)? With luck, at the end of this grant period I will be ready to return to Khovanov homology and categorification in general.
We consider computers to be outside of our field rather than a part of it, hence most of us know nothing about them. We (as a group) are happy when an undergrad writes a program to compute something for us; this done, we are happy to forget the program and use only the results. Hence a coherent uniform framework for mathematical computation does not yet exist. So every time I try to compute some complicated homology, I have to teach my computer linearity, Gaussian elimination, and tensor products practically from scratch. [...] for most mathematicians and most students of mathematics the entry barriers are way too high, their education is largely irrelevant and they get no credit for the effort. Hence so many math papers describe what amounts to be an algorithm, and so few actually implement it.
I'm a horrible student and a horrible listener. It's very difficult for me to pay attention in talks and classes; for the least reasons my mind wanders and I'm totally lost. And when I'm lost, I'm lost for good, for usually I haven't the will power to concentrate again and pick up from the last I followed.
My current computer program for computing Khovanov homology is extremely inefficient and the main reason for that is inherently mathematical - as it is, Khovanov's chain complex is just too big. An indication for that is the fact that the rank of the homology is invariably much smaller than the dimensions of the spaces of chains involved. I believe I can do a lot better by mixing some homological algebra and some sophisticated programming, and I hope to do so sometime over the grant period.
The phrase "research plan" is almost an oxymoron, for if it can be planned, it ain't research. Or at least, if it can be planned it's already half done, and hence it isn't the best of research. Thus my primary plan of mathematical research for the next few years is to follow my nose. The scents that usually attract me most are [structure, pictorial simplicity, computer assisted mathematics and weblications]