Navigation Panel: (IMAGE)(IMAGE)(IMAGE)(IMAGE) (SWITCH TO TEXT-ONLY VERSION) (IMAGE)(IMAGE) (These buttons explained below)
UNIVERSITY OF TORONTO MATHEMATICS NETWORK

Question Corner and Discussion Area

Finding the Focus of a Parabolic Dish

Asked by Nelson Siu, student, Gladstone Secondary on May 4, 1997:
I'm particular puzzled as to how I can calculate the focus of a parabolic dish. I seem to feel that it is somewhat a reverse of a locus that I've stumbled upon before. I need to find the focus because I'm using this calculation to build a parabolic listening device for my electronics class.
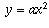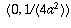It is easy to find the focus of a parabola (or the three-dimensional version, a "paraboloid") from its equation. If you draw your coordinate axes so that the origin is at the parabola's vertex and the y-axis points in the direction toward which the parabola opens, then its equation will take the form (IMAGE) for some constant a, and the focus is the point (IMAGE) . 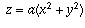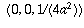Similarly for a paraboloid: if the paraboloid opens in the direction of the z-axis, it will have an equation of the form (IMAGE) , and the focus is at the point (IMAGE) .

However, these formulas are not much use in finding the focus of a particular dish you have lying around, since then you would not know the equation of the dish. Rather than trying to make measurements to determine the equation and calculate the focus from those measurements, you'd be better off finding the focus directly by experimentation (e.g., shine several parallel light beams at the dish, each parallel to the main axis of the dish, and observe where they converge). You'd likely get more accurate results that way than trying to determine the equation of the dish by measurements.

[ Submit Your Own Question ] [ Create a Discussion Topic ]
This part of the site maintained by (No Current Maintainers)
Last updated: April 19, 1999
Original Web Site Creator / Mathematical Content Developer: Philip Spencer
Current Network Coordinator and Contact Person: Joel Chan - mathnet@math.toronto.edu

Navigation Panel: (IMAGE)(IMAGE)(IMAGE)(IMAGE) (SWITCH TO TEXT-ONLY VERSION) (IMAGE)(IMAGE)

(IMAGE) Go backward to How to Draw a Circle on a Computer
(IMAGE) Go up to Question Corner Index
(IMAGE) Go forward to The Decomposition of a Drug in the Human Body
(SWITCH TO TEXT-ONLY VERSION) Switch to text-only version (no graphics)
(IMAGE) Access printed version in PostScript format (requires PostScript printer)
(IMAGE) Go to University of Toronto Mathematics Network Home Page