(These buttons explained below)
Question Corner and Discussion Area
I'm helping a 7th grader with things like: a plus times a plus equals a plus, a minus times a plus equals a minus, and a plus times a minus equals a minus. All OK. But when I tell him a minus times a minus equals a plus he says WHY? (sorry about yelling).The answer has to do with the fundamental properties of operations on numbers (the notions of "addition", "subtraction", "multiplication", and "division"). Your 7th grader's question is an important and fundamental one (which I am both surprised and sorry that he has not been able to find an answer for yet).I won't feel bad if you don't answer this. No textbook and nobody has the faintest idea. But just in case you do answer, please remember it's a 7th grader who wants to understand, not to mention yours truly.
Each number has an "additive inverse" associated to it (a sort of "opposite" number), which when added to the original number gives zero. This is in fact the reason why the negative numbers were introduced: so that each positive number would have an additive inverse.
For example, the inverse of 3 is -3, and the inverse of -3 is 3.
Note that when you take the inverse of an inverse you get the same number back again: "-(-3)" means "the inverse of -3", which is 3 (because 3 is the number which, when added to -3, gives zero). To put it another way, if you change sign twice, you get back to the original sign.
Now, any time you change the sign of one of the factors in a product, you change the sign of the product:
(-something) × (something else) is the inverse of (something) × (something else), because when you add them (and use the fact that multiplication needs to distribute over addition), you get zero.
For example,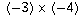 
is the inverse of 
,
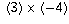because when you add
them and use the distributive law, you get
.
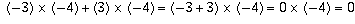So 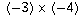is the inverse of 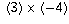, which is
itself (by similar reasoning) the inverse of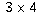 
.
Therefore, 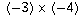is the inverse of the inverse of 12;
in other words, the inverse of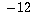 
; in other words, 12.
The fact that the product of two negatives is a positive is therefore related to the fact that the inverse of the inverse of a positive number is that positive number back again.
The answer to this question is accessible to a 7th grader (and should, in my opinion, be explained as part of every student's arithmetic classes). However, as an aside, he may be interested to know that more advanced versions of this question are studied at a university level: there is a subject called Abstract Algebra (usually only covered in a junior or senior level undergraduate university course) which studies the properties of operations on numbers in complete generality, even in contexts that have nothing to do with numbers at all. Even in such general, non-numerical contexts, the property that the product of two negative things is positive still holds.
This is a comment on your answer to the question: "Why is a negative number times a negative number a positive number?" As a volunteer teacher for a pre-algebra class of sixth graders, I addressed the same question with the following practical demonstration. I randomly handed students each a bunch of red and black checkers. I announced that the blacks (hypothetically) represented each correct answer the student had given during the class. The red checkers represented (hypothetically) their wrong answers. I told them that for each black checker, I owed them a dollar, and for each red checker they owed me a dollar. They excitedly calculated their respective balances. I then advised the students that my accounting had been wrong and I had incorrectly given each student more red checkers than I should have given them. I went from student to student, taking back (subtracting) n red checkers. After each example, I had the student recalculate their balance. For each red checker (-$1) subtracted, the students realized their balance increased by $1. After just one example, all the students cheered in unison with the joy of understanding subtracting negative numbers. I then subtracted 2 red checkers three times from the next student. Again the students cheered realizing that subtracting 2 red checkers three times was like adding six to the balance sheet. They then understood thatThank you for your comments; I have placed them as followup comments to the question.. After this demonstration, students used negative numbers in their algebra with understanding.
From my experience at Cosumnes River Elementary School Rancho Murieta, CA Ms. Lung's sixth-grade class of 1996
Do you have a place to make comments like these?
Buzz Breedlove breedlov@calweb.com
About that question: why is a negative number times a negative number equal to a positive number? I think about addition when multiplying. That isThis is essentially the same explanation given above, just with a few steps skipped over.and therefore 4 + 4 +4 = 12, therefore,
because -(-4) - (-4) - (-4) = 12. This is my logic. Can this be proven in Abstract Algebra? Is it not an easier way to explain why
The key point in your explanation is that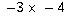 
should be the same thing as -(-4) - (-4) - (-4). The question left to
answer is, why?
Everybody can accept that taking 3 times -4 is the same thing as adding -4 together three times. The question is, why does this imply that taking -3 times -4 should be the same thing as subtracting -4 three times?
The answer is precisely because of distributivity.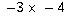
should be the negative of 
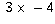: that number which, when added
to 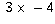, gives zero.
Since 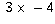is -4 added together 3 times, what you'd need to
do to it to get zero is to add 3 copies of -(-4) to cancel them out.
That is why, from the fact that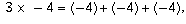 
it follows that 
Go backward to The Hypercomplex Numbers Go up to Question Corner Index Go forward to The Number Zero Switch to text-only version (no graphics) Access printed version in PostScript format (requires PostScript printer) Go to University of Toronto Mathematics Network
Home Page