© | Dror Bar-Natan: Classes: 2004-05: Math 157 - Analysis I:

(60)

Next: Class Notes for Tuesday January 4, 2005 Previous: Homework Assignment 12a

Integration

this document in PDF: Integration.pdf

The setting: $f$ bounded on $[a,b]$, $P: a=t_0<t_1<\dots<t_n=b$ a partition of $[a,b]$, $m_i=\inf_{[t_{i-1},t_i]}f(x)$, $M_i=\sup_{[t_{i-1},t_i]}f(x)$, $L(f,P)=\sum_{i=1}^n m_i(t_i-t_{i-1})$, $U(f,P)=\sum_{i=1}^n M_i(t_i-t_{i-1})$, $L(f)=\sup_P L(f,P)$, $U(f)=\inf_P U(f,P)$. Finally, if $U(f)=L(f)$ we say that ``$f$ is integrable on $[a,b]$'' and set $\int_a^b f=\int_a^b f(x)dx=U(f)=L(f)$.

Theorem 1. For any two partitions $P_{1,2}$, $L(f,P_1)\leq U(f,P_2)$.

Theorem 2. $f$ is integrable iff for every $\epsilon>0$ there is a partition $P$ such that $U(f,P)-L(f,P)<\epsilon$.

Theorem 3. If $f$ is continuous on $[a,b]$ then $f$ is integrable on $[a,b]$.

Theorem 4. If $a<c<b$ then $\int_a^b f=\int_a^c f+\int_c^b f$ (in particular, the rhs makes sense iff the lhs does).

Theorem 5. If $f$ and $g$ are integrable on $[a,b]$ then so is $f+g$, and $\int_a^b f+g = \int_a^b f + \int_a^b g$.

Theorem 6. If $f$ is integrable on $[a,b]$ and $c$ is a constant, then $cf$ is integrable on $[a,b]$ and $\int_a^b cf = c\int_a^b f$.

Theorem $\mathbf{7^a}$. If $f\leq g$ on $[a,b]$ and both are integrable on $[a,b]$, then $\int_a^b f\leq\int_a^b g$.

Theorem 7. If $m\leq f(x)\leq M$ on $[a,b]$ and $f$ is integrable on $[a,b]$ then $m(b-a)\leq\int_a^b f\leq M(b-a)$.

Theorem 8. If $f$ is integrable on $[a,b]$ and $F$ is defined on $[a,b]$ by $F(x)=\int_a^x f$, then $F$ is continuous on $[a,b]$.

        

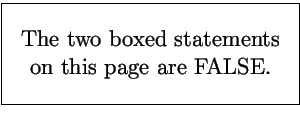
Just for fun. Why did I put these boxed statements on this page? Can they both be true? Can they both be false? If just one is true, which one must it be?

The generation of this document was assisted by L^ATEX2HTML.