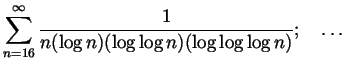
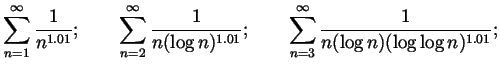
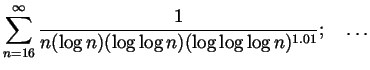
© | Dror Bar-Natan: Classes: 2004-05: Math 157 - Analysis I:

(103)

Next: Class Notes for Tuesday March 22, 2005 Previous: Solution of Term Exam 4

Homework Assignment 22

Assigned Tuesday March 22; due Friday April 1, 2PM, at SS 1071

this document in PDF: HW.pdf

Required reading. All of Spivak's Chapters 22 and 23.

To be handed in. From Spivak Chapter 23: Problems 1 (parts divisible by 4), 12, 23.

Recommended for extra practice. From Spivak Chapter 23: Problems 1 (the rest), 5, 20, 21.

In class review problem(s) (to be solved in class on Thursday March 31):

• Prove that the following sums diverge: (Hint: Use problem 20.)
  $$\sum_{n=1}^\infty \frac{1}{n}; \qquad \sum_{n=2}^\infty \frac{1}{n(\log n)}; \qquad \sum_{n=3}^\infty \frac{1}{n(\log n)(\log\log n)};$$
  $$\sum_{n=16}^\infty \frac{1}{n(\log n)(\log\log n)(\log\log\log n)}; \quad\ldots$$

• Prove that the following sums converge: (Hint: Use problem 20.)
  $$\sum_{n=1}^\infty \frac{1}{n^{1.01}}; \qquad \sum_{n=2}^\infty \f... ...g n)^{1.01}}; \qquad \sum_{n=3}^\infty \frac{1}{n(\log n)(\log\log n)^{1.01}};$$
  $$\sum_{n=16}^\infty \frac{1}{n(\log n)(\log\log n)(\log\log\log n)^{1.01}}; \quad\ldots$$

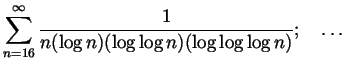
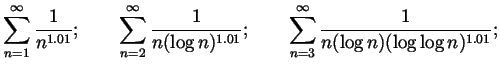
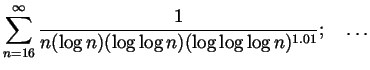
Just for fun. In this question we always assume that $a_n>0$ and $b_n>0$. Let's say that a sequence $a_n$ is ``much bigger'' than a sequence $b_n$ if $\lim_{n\to\infty}a_n/b_n=\infty$. Likewise let's say that a sequence $a_n$ is ``much smaller'' than a sequence $b_n$ if $\lim_{n\to\infty}a_n/b_n=0$. Prove that for every convergent series $\sum b_n$ there is a much bigger sequence $a_n$ for which $\sum a_n$ is also convergent, and that for every divergent series $\sum b_n$ there is a much smaller sequence $a_n$ for which $\sum a_n$ is also divergent. (Thus you can forever search in vain for that fine line between good and evil; it just isn't there).

Advertisement 1'. A short addendum to Advertisement 1 of HW21:

Date: Sun, 20 Mar 2005 21:53:48 -0500

Dr. Bar-Natan:
 
Thank you for posting our announcement on your website, the advertising
is greatly appreciated! However, a minor note: technically, this event
*does* include free food - 5 meals (not to mention a T-shirt!) are
included in the $60 registration fee, truly a fantastic bargain!   ;)
 
Cheers,
Erica Blom

The generation of this document was assisted by L^ATEX2HTML.