Dror Bar-Natan: Classes: 2002-03: Math 157 - Analysis I:

(252)

Next: Homework Assignment 23 Previous: Class Notes for the Week of March 10 (8 of 8)

Series

this document in PDF: Series.pdf

Definition. $\sum_{n=1}^\infty a_n$ is ``convergent'' or ``summable'' and $\sum_{n=1}^\infty a_n = s$ if $\sum_{n=1}^N a_n\to s$. Otherwise $\sum_{n=1}^\infty a_n$ is ``divergent''.

Claim. When the right hand sides exist, so do the left hand sides and the equalities hold:

$$\sum_{n=1}^\infty (a_n+b_n) = \sum_{n=1}^\infty a_n + \sum_{n=1}^\infty b_n,$$

$$\sum_{n=1}^\infty c\cdot a_n = c\cdot\sum_{n=1}^\infty a_n.$$

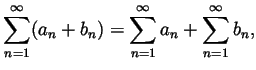
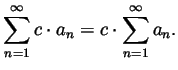
Claim. (The Boundedness Criterion) A nonnegative sequence is summable iff its partial sums are bounded.

Claim. (Cauchy's Criterion) $(a_n)$ is summable iff

$$\lim_{n,m\to\infty} a_n+\dots+a_m = 0.$$

Claim. (The Vanishing Condition) If $(a_n)$ is summable then $a_n\to 0$.

Theorem 1. (The Comparison Test) If $0\leq a_n\leq b_n$ and $\sum b_n$ converges, then so does $\sum a_n$.

Theorem 2. If $a_n>0$ and $b_n>0$ and $\lim a_n/b_n=c\neq 0$ then $\sum a_n$ converges iff $\sum b_n$ converges.

Theorem 3. (The Ratio Test) If $a_n>0$ and $\lim a_{n+1}/a_n=r$, then $\sum a_n$ converges if $r<1$ and diverges if $r>1$.

Theorem 4. (The Integral Test) If $f>0$ and $f$ is decreasing on $[1,\infty)$ and $f(n)=a_n$, then $\sum a_n$ converges iff $\int_1^\infty f$ converges.

Definition. $\sum_{n=1}^\infty a_n$ is ``absolutely convergent'' if $\sum_{n=1}^\infty \vert a_n\vert$ converges.

Theorem 5. An absolutely convergent series is convergent. A series is absolutely convergent iff the series formed from its positive terms and its negative terms are both convergent.

Theorem 6. (Leibnitz's Theorem) If $a_n$ is non-increasing and $\lim a_n=0$ then $\sum(-1)^n a_n$ converges.

Theorem 7. (skipped) If $\sum a_n$ converges but not absolutely, then for any number $s$ there is a rearrangement $(b_n)$ of $(a_n)$ so that $s=\sum b_n$.

Theorem 8. If $\sum a_n$ converges absolutely and $(b_n)$ is a rearrangement of $(a_n)$, then $\sum b_n$ also converges absolutely and $\sum a_n = \sum b_n$.

Theorem 9. If $\sum a_n$ and $\sum b_n$ converge absolutely and the sequence $c_n$ is composed of all the products of the form $a_ib_j$, then $\sum c_n = \sum a_n \cdot \sum b_n$.

The generation of this document was assisted by L^ATEX2HTML.