Homework Assignment 3
Assigned Tuesday September 28; due Friday October 8, 2PM,
at
SS 1071
Required reading. All of Spivak's Chapters 2 and
3.
To be handed in.
From Spivak Chapter 2: 1, 5.
From Spivak Chapter 3: 6, 13.
Recommended for extra practice.
From Spivak Chapter 2: 3, 4, 12, 22.
From Spivak Chapter 3: 1, 7, 21.
An extra problem: (recommended, but do not
submit) Is there a problem with the following inductive proof that all
horses are of the same color?
We assert that in all sets with precisely 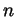 horses, all horses are of the
same color. For
horses, all horses are of the
same color. For 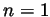 , this is obvious: it is clear that in a set with just
one horse, all horses are of the same color. Now assume our assertion is
true for all sets with
, this is obvious: it is clear that in a set with just
one horse, all horses are of the same color. Now assume our assertion is
true for all sets with 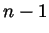 horses, and let us be given a set with
horses, and let us be given a set with 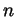 horses in it. By the inductive assumption, the first
horses in it. By the inductive assumption, the first 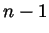 of those are of
the same color and also the last
of those are of
the same color and also the last 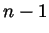 of those. Hence they are all of the
same color as illustrated below:
of those. Hence they are all of the
same color as illustrated below:
(The horses surrounded by round brackets  are all of the same
color. The horses surrounded by square brackets
are all of the same
color. The horses surrounded by square brackets ![$ [\cdots]$](img6.gif) are all of
the same color. Therefore the first and the last horses have the same
color as the ones in the middle group, and hence all horses are of the
same color.)
are all of
the same color. Therefore the first and the last horses have the same
color as the ones in the middle group, and hence all horses are of the
same color.)
Just for fun. From Spivak Chapter 2: 27, 28,
and also, more on Chapter 2, Problem 22:
- We know that if
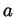 and
and 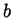 are non-negative then
are non-negative then
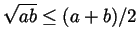 . This is the same as saying that
. This is the same as saying that
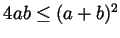 , which is the same as saying that the area of four
, which is the same as saying that the area of four 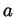 by
by 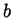 rectangles is less than or equal to the area of a square with
side
rectangles is less than or equal to the area of a square with
side 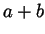 . Can you actually fit four
. Can you actually fit four 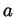 by
by 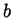 rectangles inside a
square of side
rectangles inside a
square of side 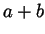 without overlaps? It's fun and not too hard.
without overlaps? It's fun and not too hard.
- We know that if
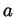 ,
, 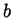 and
and 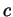 are non-negative then
are non-negative then
![$ \sqrt[3]{abc}\leq(a+b+c)/3$](img13.gif) . This is the same as saying that
. This is the same as saying that
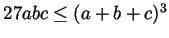 , which is the same as saying that the volume of 27
, which is the same as saying that the volume of 27
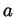 by
by 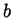 by
by 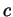 rectangular boxes is less than or equal to the volume
of a cube with side
rectangular boxes is less than or equal to the volume
of a cube with side 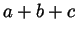 . Can you actually fit 27 such
. Can you actually fit 27 such 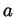 by
by 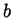 by
by
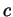 rectangular boxes inside a cube of side
rectangular boxes inside a cube of side 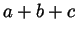 without overlaps?
This is also fun, but quite hard. You have no chance of doing it
without a physical model. Make yourself one!
without overlaps?
This is also fun, but quite hard. You have no chance of doing it
without a physical model. Make yourself one!
- The corresponding problem in 4D, involving 256 boxes of size
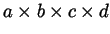 , is actually a little easier, though
trickier, than the 3D problem. Can you do it?
, is actually a little easier, though
trickier, than the 3D problem. Can you do it?
- The corresponding problem in 5D, involving 3,125 boxes of size
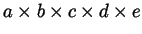 , is an open problem -- meaning
that nobody knows how to solve it. Can you?
, is an open problem -- meaning
that nobody knows how to solve it. Can you?
The generation of this document was assisted by
LATEX2HTML.
Dror Bar-Natan
2004-09-27
![]() horses, all horses are of the
same color. For
horses, all horses are of the
same color. For ![]() , this is obvious: it is clear that in a set with just
one horse, all horses are of the same color. Now assume our assertion is
true for all sets with
, this is obvious: it is clear that in a set with just
one horse, all horses are of the same color. Now assume our assertion is
true for all sets with ![]() horses, and let us be given a set with
horses, and let us be given a set with ![]() horses in it. By the inductive assumption, the first
horses in it. By the inductive assumption, the first ![]() of those are of
the same color and also the last
of those are of
the same color and also the last ![]() of those. Hence they are all of the
same color as illustrated below:
of those. Hence they are all of the
same color as illustrated below:
![$\displaystyle \left(\begin{array}{c}
\includegraphics[width=0.6in]{RockingHorse...
...n{array}{c}
\includegraphics[width=0.6in]{RockingHorse.eps}
\end{array}\right] $](img4.gif)