November 1997 Presentation Topic (continued)

Some Tools to Solve the Problems

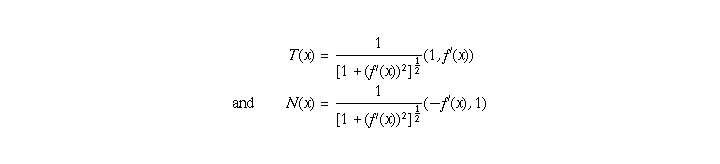
are, respectively, tangent and normal to S at the point (x,f(x)). See figure 5.

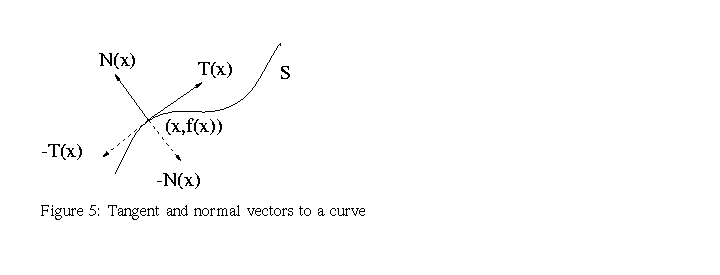
A parametrization of a curve 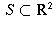is a map from an interval 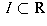onto 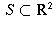given by

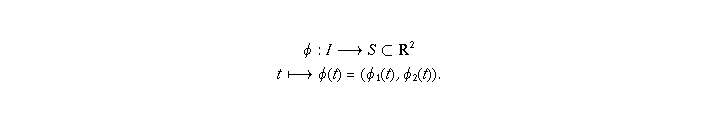
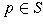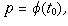
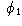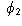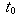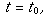The curve S is said to be smooth at a point if and are smooth at and the tangent vector does not vanish at i.e.,

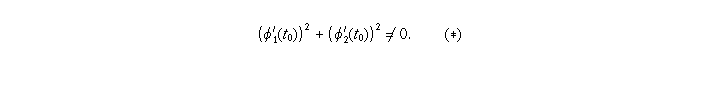
When (*) fails, the curve S has a singularity at p.

Example: The curve 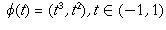has a singularity at (0,0). Indeed, the tangent vector is given by 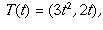which vanishes at t=0. If we set 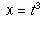and 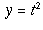we see that this curve is given by 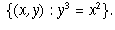This curve is called a cubic parabola, and this type of singularity a cusp. The graph of this curve can be seen in figure 6.

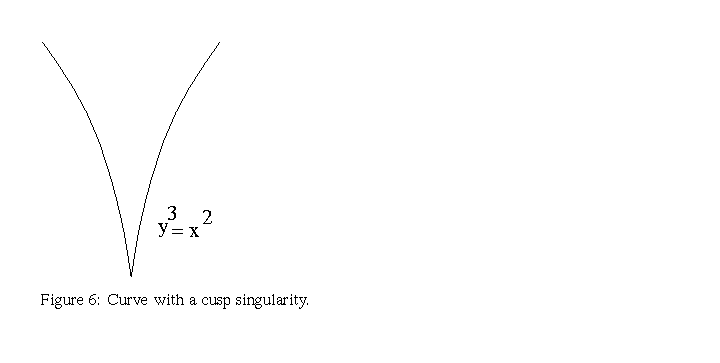
Go backward to Problems
Go up to Introduction and Contents
Go forward to Solutions to the problems
Switch to text-only version (no graphics)
Access printed version in PostScript format (requires PostScript printer)
Go to SIMMER Home Page
Go to The Fields Institute Home Page
Go to University of Toronto Mathematics Network Home Page