Question Corner and Discussion Area
Question Corner and Discussion Area
Hi!The series you have described is not a geometric series. It is an example of a more general class of series called power series, which are of the formMy question is about geometric series. I read about the one that you solved, but this one is a little bit different :
What is the sum from i = 0 to infinity of (x^i)(i^2)?
Thanks.
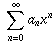 where the coefficients
where the coefficients
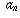 don't depend on the variable x. In your example,
don't depend on the variable x. In your example,
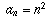 .
.
A key fact about power series is that, if the series converges on an interval of the form |x| < R, then it "converges uniformly" on any closed subinterval of that interval. I won't attempt to explain what that means, but will mention instead an important consequence (which is not always true for series that are not power series): the series can be integrated and differentiated term by term, in the sense that, if you define
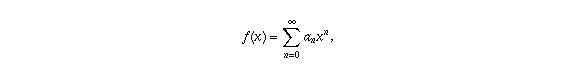
then
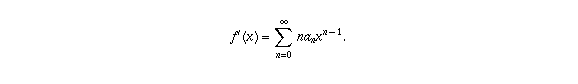
This means that, if you start with the geometric series 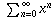 which is
known to converge to 1/(1-x) when |x| < 1 (as described in
the answer to another question), the following is
true for all |x| < 1 by differentiating both sides of the equation:
which is
known to converge to 1/(1-x) when |x| < 1 (as described in
the answer to another question), the following is
true for all |x| < 1 by differentiating both sides of the equation:
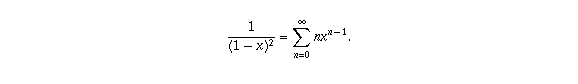
If you multiply both sides by x you get something close to what you want:
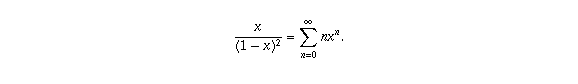
Differentiating both sides again and multiplying by x again gives you what you want:

Therefore, your series converges to 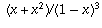 , provided
|x| < 1. (If |x| > 1, it diverges).
, provided
|x| < 1. (If |x| > 1, it diverges).
This particular technique will, of course, work only for this specific example, but the general method for finding a closed-form formula for a power series is to look for a way to obtain it (by differentiation, integration, etc.) from another power series whose sum is already known (such as the geometric series, or a series you can recognize as the Taylor series of a known function).
Most series don't have a closed-form formula, but for those that do, the above general strategy usually helps one to find it.
![]() Go backward to An Infinitely Recurring Square Root
Go backward to An Infinitely Recurring Square Root![]() Go up to Question Corner Index
Go up to Question Corner Index![]() Go forward to The Sum of the Geometric Series 1 + 1/2 + 1/4 + ...
Go forward to The Sum of the Geometric Series 1 + 1/2 + 1/4 + ...![]() Switch to text-only version (no graphics)
Switch to text-only version (no graphics)![]() Access printed version in PostScript format (requires PostScript printer)
Access printed version in PostScript format (requires PostScript printer)![]() Go to University of Toronto Mathematics Network
Home Page
Go to University of Toronto Mathematics Network
Home Page