(These buttons explained below)
Question Corner and Discussion Area
Could you please give us a formula for figuring out what pi is in base 6? We have the numbers 3,0,5,0,3,3,0,0,5,1,4,5, etc. but we do not know exactly how to obtain these numbers.Digits of pi are generally calculated by representing pi as the sum of an infinite series, then evaluating that sum to as many terms as are necessary to get the desired number of digits.
One way is to use the formula Arctan(x) = x - x^3 + x^5/5 - x^7/7 + . . . ,
which is valid for 
. Plugging in x=1 is not much use
because, although you get a formula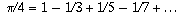 
, the series converges very slowly and you have to take
thousands of terms to get even a few digits of accuracy.
A faster way is to use a formula such as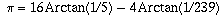 
. You evaluate each of these arctangents using the above
series, taking enough terms of the series to get you to within the accuracy
you desire. In your case, you would do the arithmetic in base 6 to get
digits of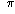 
in base 6.
Another, more recent, series is
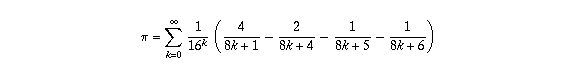
which has the advantage that in base 16 there turns out to be a clever
way to use this formula to calculate far-out digits of 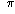without
having to do high-precision arithmetic and without having to compute all
the earlier digits first. That's only a help in base 16 and a few other
bases, though.
For example, if you were to use this latter series to calculate the first
five base-6 digits of 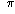, you would say that you want 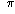to
within an accuracy of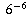 
(which is about 0.00002).
Note that the k-th term in the above
series is smaller than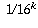 
, so if you add up the terms from k=n+1
onwards the most you will get is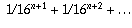 
which,
by the formula for summing a geometric series, is
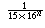. This, then, is the maximum error you could
possibly introduce by taking only the first n terms of the series.
If you take n=3, the above estimate gives you an error of at most 0.0000163 which is smaller than your desired accuracy. Therefore, all you need to do is take the first three terms of the series. You would evaluate the terms when k=0, when k=1, when k=2, and when k=3 and add them up, doing the calculations in base 6.
Go backward to Largest Possible Number? Go up to Question Corner Index Go forward to Tetrahedral and 4-Tetrahedral Numbers Switch to text-only version (no graphics) Access printed version in PostScript format (requires PostScript printer) Go to University of Toronto Mathematics Network
Home Page