(These buttons explained below)
Question Corner and Discussion Area
I want to know how to construct a pentagon. I have done it before, but I have forgotten how. I remember it is similar to constructing a hexagon, but a bit more difficult. Thanks.There are several ways to do it. Unfortunately we are very short-staffed right now and cannot spare the resources to hunt down the easiest and most elegant construction. However, the following method will work:
Constructing a pentagon is equivalent to dividing a circle (a full 360
degrees) up into five equal parts (angle 72 degrees each). The cosine of
72 degrees is 
(this can be found by starting with the
equation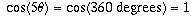 
,
using trigonometric identities to write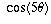 
as a polynomial
in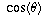 
, factoring and solving the resulting polynomial equation
for ).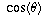
Therefore, this angle of 72 degrees can be constructed by building a
right-angled triangle whose hypotenuse is 4 and whose adjacent side is
of length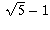 
. This latter length can be constructed by taking
hypotenuse of a right triangle whose other sides have lengths 1 and 2,
and subtracting length 1 from it.
The following procedure uses this idea to construct a pentagon:
Start with a circle C, with centre point O. Let P be a point on C. Draw the perpendicular bisector L to segment OP (bisecting it at point Q). Construct the midpoint R of OQ. (RQ is going to be our unit length).
With centre Q and radius RQ, draw an arc intersecting L at point
S. Draw segment OS.
(This is the hypotenuse of a right triangle OQS whose other sides have
length 1 and 2, so OS has length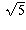 
).
With centre S and radius RQ (= QS), draw an arc intersecting OS at point
T. (Now OT has length ).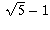
Construct the line passing through point T at right angles to OT. Let it intersect the circle C at point U.
Now the triangle OTU has hypotenuse of length OU = radius of C = 4,
and side length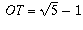 
. Therefore, angle UOT is 72 degrees.
Extend segment OT past S until it meets the circle C at point V;
you have now constructed two vertices (U and V) of the pentagon.
To construct the remaining vertices: with centre V and radius UV draw an arc intersecting C at point W. With centre W and the same radius, draw an arc intersecting C at point X. Finally, with centre X and the same radius, draw an arc intersecting C at point Y. UVWXY will be a pentagon.
There are probably much more efficient ways to do it, but the above procedure will certainly work, for the reasons described. The procedure is illustrated below:
Go backward to Do Parallel Lines Meet At Infinity? Go up to Question Corner Index Go forward to The Three Classical Impossible Constructions of Geometry Switch to text-only version (no graphics) Access printed version in PostScript format (requires PostScript printer) Go to University of Toronto Mathematics Network
Home Page