Question Corner and Discussion Area
Question Corner and Discussion Area
I am an artist and have a project where I have to construct a 4 sided pyramid out of glass for a garden fountain. Each of the four triangles have a base of 30.5 inches and a height of 46 inches. I need to know what degree of miter to put on the edges of the uprights of the triangles so that it will all fit together nicely. Thanks for any help you can give me. I have come up with 54.125 degrees on each of the upright edges. I am sure there must be a formula but I cannot find it. Thanks again.Let's denote the measure of the angle of the miter by A. The angle of the miter needs to be half the angle formed by two faces of the pyramid, so the angle between these faces is 2A. If we find this angle, we can divide by 2 to get A.
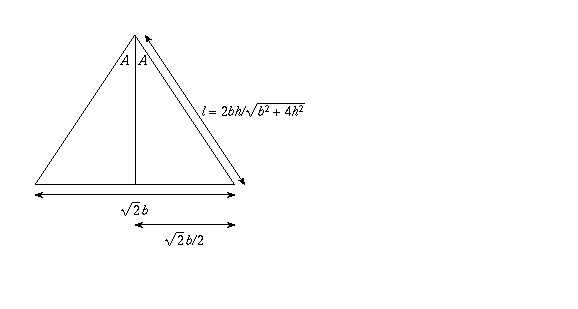
The way to find the angle between these two adjacent triangular faces is to think of the common edge of these triangles as their bases and to construct two line segments, perpendicular to this edge, corresponding to their altitudes. The angle that these line segments make when joined together is the same as the angle between the faces, which is 2A. This is illustrated below (the first picture shows the triangular faces separate, the second shows them assembled into the pyramid).
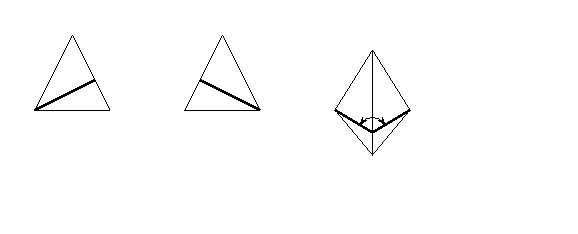
Now we need to compute the angle at which these two line segments meet. To do this we need two things: (a) their length (they are of equal length), and (b) the length of the third side of the triangle formed by these two line segments.
Let's find length (b) first. The third side
(running from the end of the second line
segment back to the start of the first) is a diagonal of the base of the
pyramid. Since the base of the pyramid is a square, whose sides are the
bases of the triangles, the length of this diagonal is 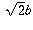 where b denotes the base length of each triangle.
where b denotes the base length of each triangle.
Length (a) be computed by finding the area of a face of the pyramid in two ways. One way is to use the standard base and height measurements (thinking of the bottom edge as the base). The area is 1/2 bh, where h is the height and b the length of the base.
The other way is to think of one of the slanted edges of the triangle as
the "base", with our unknown line segment length being the "height".
The length of the edge can be computed, using
the Pythagorean theorem, to be 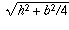 .
If we let l denote the length of our unknown side,
we can see that the area is
.
If we let l denote the length of our unknown side,
we can see that the area is 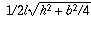 .
Since we also know that the area is 1/2 bh, we can equate these two
values and solve for l, which gives
.
Since we also know that the area is 1/2 bh, we can equate these two
values and solve for l, which gives
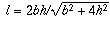 .
.
We now know that our two line segments, which meet at an angle of measure
2A, have length 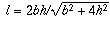 and form two sides of a
triangle whose third length is
and form two sides of a
triangle whose third length is 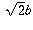 . As illustrated below,
that means the angle A is part of a right-angled triangle whose hypotenuse
has length
. As illustrated below,
that means the angle A is part of a right-angled triangle whose hypotenuse
has length 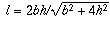 and whose opposite side has
length
and whose opposite side has
length 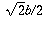 .
.
Thus the angle A is given by
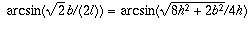 .
Plugging in our values of h = 46 and b = 30.5
we find that A is approximately 48.155 degrees.
.
Plugging in our values of h = 46 and b = 30.5
we find that A is approximately 48.155 degrees.
![]() Go backward to The Decomposition of a Drug in the Human Body
Go backward to The Decomposition of a Drug in the Human Body![]() Go up to Question Corner Index
Go up to Question Corner Index![]() Go forward to Applications of Polynomial Factorization
Go forward to Applications of Polynomial Factorization![]() Switch to text-only version (no graphics)
Switch to text-only version (no graphics)![]() Access printed version in PostScript format (requires PostScript printer)
Access printed version in PostScript format (requires PostScript printer)![]() Go to University of Toronto Mathematics Network
Home Page
Go to University of Toronto Mathematics Network
Home Page