Question Corner and Discussion Area
Question Corner and Discussion Area
Can you give one example of a directly measurable quantity which has an imaginary number in it? In all cases I've seen you either ignore the imaginary root or square it into a real quantity.For your first question, here are some examples (see also the "answers and explanations" section of the web site, as well as an earlier question in the Question Corner area):Second, don't imarginary numbers arise because we ask that multiplication is communitive, i.e. A × B=B × A, but if we dropped this rule, and defined multiplication as 1 × 1=1, -1 × -1=-1, -1 × 1=-1, 1 × -1=1, then I believe we would lose the imaginary terms, but be stuck with a messier (similar to operator algebra) mathematics.
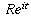 .
.
A field F that enters medium M will emerge as F times M (where this product is by the laws of complex multiplication). It is this fact that justifies measuring the entire field by a single complex number rather than taking its electric and magnetic components separately and measuring each by a real number, for in the latter case you wouldn't have a way to multiply.
As for your second question: commutativity is not the issue. More important is the fact that multiplication has to be related to addition by the distributive law.
There are plenty of non-commutative extensions of the reals (such as the quaternions), but any that satisfy the distributive law and are complete with respect to root-taking must of necessity contain the complex numbers as a subset.
Your example is simply a function from pairs of real numbers to real numbers for which there happens to exist a "root" r of -1 in the sense that f(r,r)=-1. There are plenty of such functions, but unless they are linear and distribute over addition, they don't provide the structure needed for a notion of "multiplication".
For instance, in your example you would have 1 × (1 + (-1)) = 1 × 0 = 0, but (1 × 1) + (1 × (-1) = 1 + 1 = 2.
Non-commutative multiplication is messier but still useful, but an operation that is not commutative, not associative, and doesn't distribute over addition cannot really play the part of multiplication in any reasonable mathematical theory.
![]() Go backward to Complex Numbers in Real Life
Go backward to Complex Numbers in Real Life![]() Go up to Question Corner Index
Go up to Question Corner Index![]() Go forward to Geometry and Imaginary Numbers
Go forward to Geometry and Imaginary Numbers![]() Switch to text-only version (no graphics)
Switch to text-only version (no graphics)![]() Access printed version in PostScript format (requires PostScript printer)
Access printed version in PostScript format (requires PostScript printer)![]() Go to University of Toronto Mathematics Network
Home Page
Go to University of Toronto Mathematics Network
Home Page