Question Corner and Discussion Area
Question Corner and Discussion Area
Hello,Geometric progressions have been found on Babylonian tablets dating back to 2100 BC. Arithmetic progressions were first found in the Ahmes Papyrus which is dated at 1550 BC. The names for these notions, however, seem to have taken considerably longer. In some cases there was no standard for how to refer to them (even the term progression was not necessarily a standard).My question is not an actual problem, but it's been puzzling me and I haven't found an accurate answer yet. Maybe you could help me.
Why are arithmetic sequences actually called arithmetic? What about them makes it arithmetic? What about geometric sequences. . . why aren't they called arithmetic? Don't they contain arithmetic in them?
Also why is some sequences both arithmetic and geometric, like 2,2,2,2,2,2,. . . ? The obvious answer is it that one has a common difference and the other a common ratio, but it's not completly clear to me. I believe that arithmetic sequences are called that because of thier similarity to arithmetic progressions, but it doesn't completly explain to me the real concept about it.
Please reply A.S.A.P. Thank you for your time in answering my question and hope it doesn't trouble you!! :-)
Yours Truly,
Flavia Fayet
The closest I can come to the reasoning behind the names is that each
term in a geometric (arithmetic) sequence is the geometric (arithmetic)
mean
of it's successor and predessor.
The rationale behind the names of these means is a bit more clear:
if we view the quantities A and B as the lengths of the sides of a
rectangle,
then the geometric mean 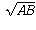 is the length
of the sides of a square
having
the same area as this rectangle. This was viewed in those days as a very
geometric problem: finding the dimensions of a square having
the same area as a given figure (in this case, rectangle).
is the length
of the sides of a square
having
the same area as this rectangle. This was viewed in those days as a very
geometric problem: finding the dimensions of a square having
the same area as a given figure (in this case, rectangle).
Although the arithmetic mean (A+B)/2 can also be interpreted geometrically (it is the length of the sides of a square having the same perimeter as the rectangle), lengths were viewed more as arithmetical concepts (because it's easy to handle lengths by ordinary addition and subtraction, without having to think about two-dimensional concepts such as area).
You are, of course, perfectly right when you say that both concepts involve arithmetic. It is also true that both concepts can be interpreted geometrically. Nevertheless, in ancient times one was viewed much more geometrically than the other, hence the names. I hope the above paragraphs shed some light on why that was so.
![]() Go backward to Which U.S. President Re-Proved the Pythagorean Theorem?
Go backward to Which U.S. President Re-Proved the Pythagorean Theorem?![]() Go up to Question Corner Index
Go up to Question Corner Index![]() Go forward to Origin of the Notation for Slope
Go forward to Origin of the Notation for Slope![]() Switch to text-only version (no graphics)
Switch to text-only version (no graphics)![]() Access printed version in PostScript format (requires PostScript printer)
Access printed version in PostScript format (requires PostScript printer)![]() Go to University of Toronto Mathematics Network
Home Page
Go to University of Toronto Mathematics Network
Home Page