$\renewcommand{\Re}{\operatorname{Re}}$ $\renewcommand{\Im}{\operatorname{Im}}$ $\newcommand{\erf}{\operatorname{erf}}$ $\newcommand{\dag}{\dagger}$ $\newcommand{\const}{\mathrm{const}}$ $\newcommand{\arcsinh}{\operatorname{arcsinh}}$

1D Wave equation: IBVP-Visualization on the half-line

For two wave equations

\begin{align*} &u_{tt}-c_1^2u_{xx}=0 && \text{as } x>0,\\[3pt] &u_{tt}-c_2^2u_{xx}=0 && \text{as } x<0, \end{align*}

intertwined through boundary conditions \begin{align*} u|_{x=0^+}&=u|_{x=0^-}\\[3pt] u_x|_{x=0^+}&=k u_x|_{x=0^-} \end{align*} with $k>0$.

In this case

1. $u(x,t)= \phi (t+c_1^{-1} x) +\psi (t-c_1^{-1} x)$ for $x >0$ and
2. $u(x,t)=\chi(t+c_2^{-1} x)$ for $x< 0$

with $\psi(t) =-\beta \phi(t)$, $\chi(t)=\alpha \phi(t)$, $\alpha = 2c_2(kc_1+c_2)^{-1}$, $\beta=(kc_1-c_2)(kc_1+c_2)^{-1}$.

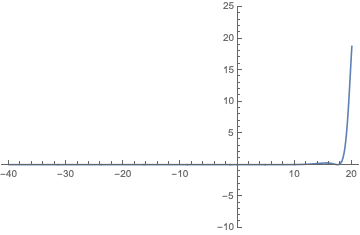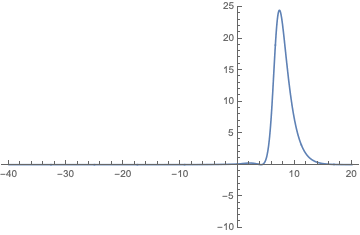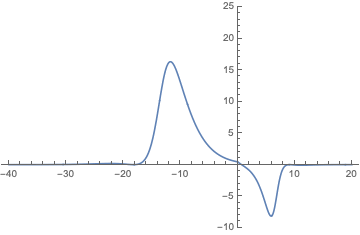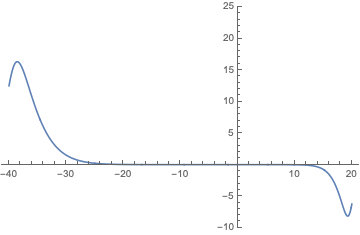
Then we have two outgoing waves: reflected wave $\psi (t-c_1^{-1} x)$ and refracted wave $\chi(t+c_2^{-1} x)$.