| © | Dror Bar-Natan: Classes: 2004-05: Math 157 - Analysis I: | (78) |
Next: Plots of the trigonometric functions
Previous: Class Notes for Thursday January 27, 2005 |
On Term Exam 3. It will take place, as scheduled, during the tutorials on Monday February 7th. You will have an hour and 50 minutes to solve around 5 questions, with no choice questions. The material is everything covered in class until Tuesday February 1st (and not including Thursday February 3rd), including everything in the relevant chapters (12-15) of Spivak's book (though not including the appendices to these chapters). The material in chapters 1-11 is not officially included, though, of course, what chance have you got answering questions about the fundamental theorems of calculus (say), if you aren't yet absolutely fluent with derivatives? Some questions will be taken straight from class, some straight from homework, and some will be fresh. Calculators will be allowed but will not be useful beyond emotional support; no devices that can display text will be allowed. Good luck!
Preparing for Term Exam 3.
An often-asked question is ``Do we need to know proofs?''. The answer is Absolutely. Proofs are often the deepest form of understanding, and hence they are largely what this class is about. The ones I show in class are precisely those that I think are the most important ones, thus they are the ones you definitely need to know.
Required reading. All of Spivak's chapters 15 and 18.
To be handed in. From Spivak Chapter 18: 1 (ii,
v, ix), 4 (odd parts), 7 (odd parts, though in (c),
``
![]() '' should be ``
'' should be ``
![]() ''), 8 (odd parts), 21.
''), 8 (odd parts), 21.
Recommended for extra practice. From Spivak Chapter 18: 1 (the rest), 4 (even parts), 6, 7 (even parts), 8 (even parts), 18, 34, 47 (a-d), 49.
In class review problem(s) (to be solved in class next Tuesday). Chapter 18 problem 47e: Arrange each of the following sets of functions in increasing order of growth:
Aside. Here's a short
Mathematica session that computes an
approximation of the number ![]() for which
for which
![]() :
:
In[1]:= s = 0; t = 1; dt = 0.000001; In[2]:= While[(s += dt/t) < 1, t += dt]; t Out[2]= 2.71828
For your information, an email I got a couple days ago:
Date: Sun, 30 Jan 2005 21:24:54 -0500 (EST) From: pi movie <pimovie@yahoo.ca> Subject: pi documentary promo Hello, My name is Katie Cooper and I'm a Film Student at Humber College. My thesis project "easy as pi" is a documentary all about "pi day", the annual math event occuring every 3rd month (March), 14th day @ 1:59 pm (hence the first digits, 3.14159). I'm in the post-production and promotion phase of the project and am hoping you can help me out as I am looking for math students or professors or anyone who wants to recite digits of pi for a contest Feb.15, 2005. If you could forward the below message to some students or let me know where the math building is located so I can post some promo information. The show will repeat on the radio for 4 weeks right up to pi day 2005. Please read below: Tuesday, February the 15th from 8-10pm broadcasting live out of University of Toronto, Totally Sweet Radio is doing a show promoting "easy as pi" (my documentary) and of course pi day 2005 coming up March 14th! We are looking for people who can recite digits of pi and are up for a competition! Ryan from my doc will be reciting and we need some competitors live in the studio to go against him! If you are interested in competing, contact myself, Katie at pimovie@yahoo.ca thanks! Katie Cooper director/producer --easy as pi--
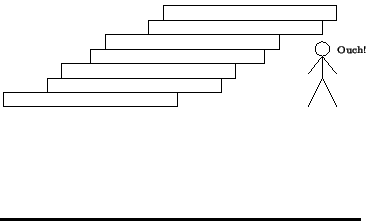
Just for fun. How far can you reach by stacking
up ![]() identical domino pieces, before your tower will lean over and fall?
(You may slide each piece a different amount, if you wish).
identical domino pieces, before your tower will lean over and fall?
(You may slide each piece a different amount, if you wish).