Dror Bar-Natan: Classes: 2002-03: Math 157 - Analysis I:

(262)

Next: Solution of Term Exam 4 Previous: Class Notes for the Week of March 17 (8 of 8)

Term Exam 4

University of Toronto, March 24, 2003

this document in PDF: Exam.pdf

Solve the following 5 problems. Each is worth 20 points although they may have unequal difficulty. Write your answers in the space below the problems and on the front sides of the extra pages; use the back of the pages for scratch paper. Only work appearing on the front side of pages will be graded. Write your name and student number on each page. If you need more paper please ask the tutors. You have an hour and 50 minutes.

Allowed Material: Any calculating device that is not capable of displaying text.

Good Luck!

Problem 1. Is there a non-zero polynomial $p(x)$ defined on the interval $[0,\pi]$ and with values in the interval $[0,\frac12)$ so that it and all of its derivatives are integers at both the point 0 and the point $\pi$? In either case, prove your answer in detail. (Hint: How did we prove the irrationality of $\pi$?)

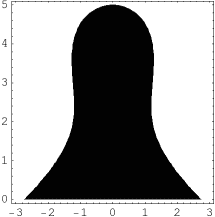Problem 2. Compute the volume $V$ of the ``Black Pawn'' on the right -- the volume of the solid obtained by revolving the solutions of the inequalities $4x^2\leq y+3-(y-3)^3$ and $y\geq 0$ about the $y$ axis (its vertical axis of symmetry). (Check that $5+3-(5-3)^3=0$ and hence the height of the pawn is $5$).

Problem 3.

1. Compute the degree $n$ Taylor polynomial $P_n$ of the function $f(x)=\frac{1}{1-x}$ around the point 0.
2. Write a formula for the remainder $f-P_n$ in terms of the derivative $f^{(n+1)}$ evaluated at some point $t\in[0,x]$.
3. Show that at least for very small values of $x$, $f(x)=\lim_{n\to\infty}P_n(x)$.

Problem 4.

1. Prove that if $\lim_{n\to\infty} a_n=l$ and the function $f$ is continuous at $l$, then $\lim_{n\to\infty}f(a_n)=f(l)$
2. Let $b>1$ be a number, and define a sequence $a_n$ via the relations $a_1=1$ and $a_{n+1}=\frac12(a_n+b/a_n)$ for $n\geq 1$. Assuming that this sequence is convergent to a positive limit, determine what this limit is.

Problem 5. Do the following series converge? Explain briefly why or why not:

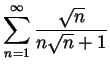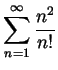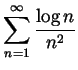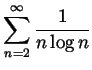1. $$\sum_{n=1}^\infty \frac{n}{2n+1}$$.
2. $$\sum_{n=1}^\infty \frac{\sqrt{n}}{n\sqrt{n}+1}$$.
3. $$\sum_{n=1}^\infty \frac{n^2}{n!}$$.
4. $$\sum_{n=1}^\infty \frac{\log n}{n^2}$$.
5. $$\sum_{n=2}^\infty \frac{1}{n\log n}$$.

The generation of this document was assisted by L^ATEX2HTML.