Table of Contents

1. Arthur Huang spoke on the Rogers-Varga nonelliptic mass concentration result
2. Averaging over hypersurfaces as quoted in Rogers-Vargas
3. References
4. Study strategy
   1. The Hardy-Littlewood Maximal Theorem
   2. Theorems on Spherical Means
   3. One can then start to unpackage the Sogge-Stein theorems and appreciate the generality of the hypotheses appearing therein.

(MultiMarkDown Source Code generating this file)

Arthur Huang spoke on the Rogers-Varga nonelliptic mass concentration result ↩

Yesterday, Arthur Huang continued discussing the article of Rogers-Vargas establishing mass concentration for nonelliptic cubic NLS if blowup occurs. Like the antecedent work by Bourgain, this advance is based on refinements of the Strichartz inequality. There was some discussion in the seminar along this direction.

• Arthur wrote to me last night:

from as.huang@utoronto.ca to “J. Colliander” date Tue, Nov 2, 2010 at 5:43 PM

Hi prof,

Thank you for helping me clearifying several points in the seminar! Due to the lack of background, sometimes I thought I understood but in fact I haven’t really make a cristal clear sence of that… throughout this talk I really learned a lot! Especially what you explained about Sogge&Stein theorem pointed me a way to get much better understanding about integral operators.

Can you tell me exactly which book did you mean by Sogge? I hope to get a thorough understanding of that theorem.

Best, Arthur

• These notes begin a conversation in that direction.

Averaging over hypersurfaces as quoted in Rogers-Vargas ↩

The theorem quoted in RV and attributed to Sogge-Stein we discussed is:

References ↩

• [13] Sogge-Stein 1990
• Sogge-Stein 1986
• Sogge-Stein 1985

Study strategy ↩

If you really want to get into the background on this topic, I suggest you first learn

The Hardy-Littlewood Maximal Theorem ↩

These papers are inspired by the classical study of the Hardy-Littlewood maximal operator which arises naturally in the study of differentiation properties of the Lebesgue integral. For a rapid discussion of that topic, one might consult the text book of Sogge. There is also a thorough discussion of this topic in the famous textbooks of Stein and Stein-Weiss.

Theorems on Spherical Means ↩

• Stein 1976

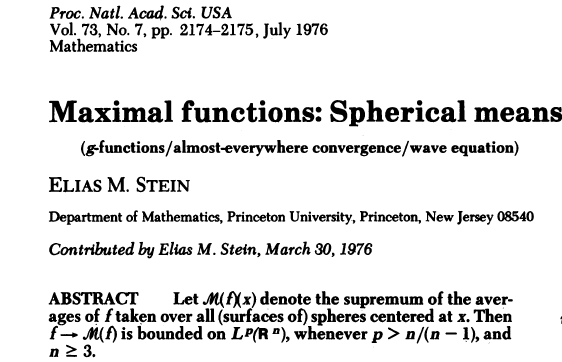
Observe that this theorem only applies in demensions $n \geq 3$. This theorem was extended to $n = 2$ (using different ideas) by Bourgain in the mid-80s.

• Bourgain 1986

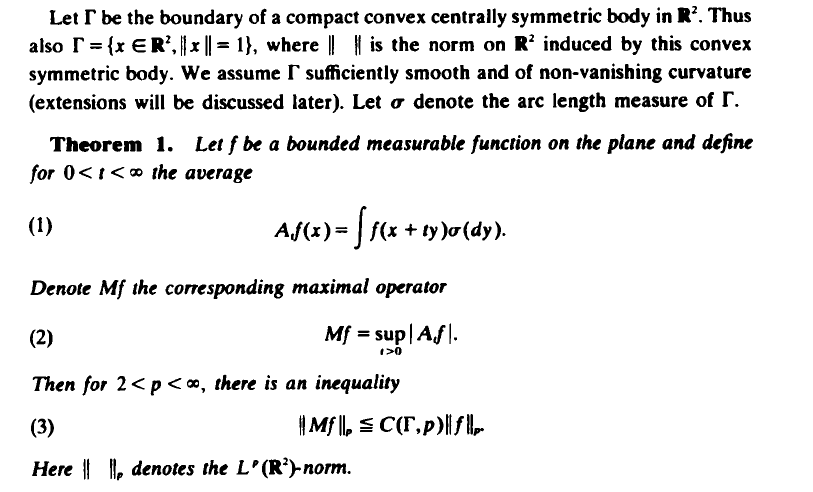
A flurry of activity, including the contributions of Sogge-Stein mentioned above, in harmonic analysis followed Bourgain’s work.

One can then start to unpackage the Sogge-Stein theorems and appreciate the generality of the hypotheses appearing therein. ↩